| Trigonometry |
|---|
 |
| Reference |
| Laws and theorems |
| Calculus |
The exsecant (exsec, exs) and excosecant (excosec, excsc, exc) are trigonometric functions defined in terms of the secant and cosecant functions. They used to be important in fields such as surveying, railway engineering, civil engineering, astronomy, and spherical trigonometry and could help improve accuracy, but are rarely used today except to simplify some calculations.
Exsecant
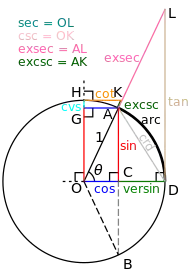
The exsecant,[2][3][4][5][6][7][8][9] (Latin: secans exterior[10][11][12][13]) also known as exterior, external,[14][15][16][17] outward or outer secant and abbreviated as exsec[18][19][20][21] or exs,[22] is a trigonometric function defined in terms of the secant function sec(θ):[23]
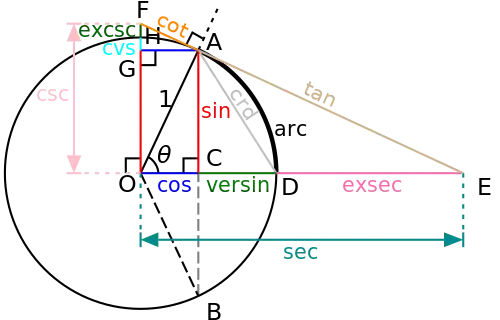
The name exsecant can be understood from a graphical construction of the various trigonometric functions from a unit circle, such as was used historically. sec(θ) is the secant line OE, and the exsecant is the portion DE of this secant that lies exterior to the circle (ex is Latin for out of).
Excosecant
A related function is the excosecant[5][24] or coexsecant,[25][18][26] also known as exterior, external,[17] outward or outer cosecant and abbreviated as excosec, coexsec,[14][18][26] excsc[5][24] or exc,[22] the exsecant of the complementary angle:[24]
Usage
Important in fields such as surveying,[8] railway engineering[5] (for example to lay out railroad curves and superelevation), civil engineering, astronomy, and spherical trigonometry up into the 1980s, the exsecant function is now little-used.[8][23] Mainly, this is because the broad availability of calculators and computers has removed the need for trigonometric tables of specialized functions such as this one.[8]
The reason to define a special function for the exsecant is similar to the rationale for the versine: for small angles θ, the sec(θ) function approaches one, and so using the above formula for the exsecant will involve the subtraction of two nearly equal quantities, resulting in catastrophic cancellation. Thus, a table of the secant function would need a very high accuracy to be used for the exsecant, making a specialized exsecant table useful. Even with a computer, floating point errors can be problematic for exsecants of small angles, if using the cosine-based definition. A more accurate formula in this limit would be to use the identity:[3][4][17]
or[17]
Prior to the availability of computers, this would require time-consuming multiplications.
The exsecant function was used by Galileo Galilei in 1632 already, although he still called it segante (meaning secant).[27][28][29][30] The Latin term secans exterior was used since at least around 1745.[10][11][12][13] The usage of the English term external secant and the abbreviation ex. sec. can be traced back to 1855 the least, when Charles Haslett published the first known table of exsecants.[1][31] Variations such as ex secant and exsec were in use in 1880,[14] and exsecant was used since 1894 the least.[2]
The terms coexsecant[25] and coexsec[2] can be found used as early as 1880 as well[2][25] followed by excosecant since 1909.[5] The function was also utilized by Albert Einstein to describe the kinetic energy of fermions.[29][30]
Mathematical identities
Derivatives
Integrals
Inverse functions
The inverse functions arcexsecant[26] (arcexsec,[5][26] aexsec,[32][33] aexs, exsec−1) and arcexcosecant (arcexcosec, arcexcsc,[5] aexcsc, aexc, arccoexsecant, arccoexsec, excsc−1) exist as well:
Other properties
Derived from the unit circle:
The exsecant function is related to the tangent function by[23]
In analogy, the excosecant function is related to the cotangent function by
The exsecant function is related to the sine function by
In analogy, the excosecant function is related to the cosine function by[30]
The exsecant and excosecant functions can be extended into the complex plane.[21]
See also
- Trigonometric identities – Equalities that involve trigonometric functions
- Versine – 1 minus the cosine of an angle
- Chord – Geometric line segment whose endpoints both lie on the curve
- Incircle and excircles of a triangle – Circles tangent to all three sides of a triangle
- Exponential minus 1 – Mathematical function, denoted exp(x) or e^x
- Natural logarithm plus 1 – Logarithm to the base of the mathematical constant e
References
- 1 2 Haslett, Charles (September 1855). Hackley, Charles W. (ed.). The Mechanic's, Machinist's, Engineer's Practical Book of Reference: Containing tables and formulæ for use in superficial and solid mensuration; strength and weight of materials; mechanics; machinery; hydraulics, hydrodynamics; marine engines, chemistry; and miscellaneous recipes. Adapted to and for the use of all classes of practical mechanics. Together with the Engineer's Field Book: Containing formulæ for the various of running and changing lines, locating side tracks and switches, &c., &c. Tables of radii and their logarithms, natural and logarithmic versed sines and external secants, natural sines and tangents to every degree and minute of the quadrant, and logarithms from the natural numbers from 1 to 10,000. New York, USA: James G. Gregory, successor of W. A. Townsend & Co. (Stringer & Townsend). Retrieved 2017-08-13.
[…] Still there would be much labor of computation which may be saved by the use of tables of external secants and versed sines, which have been employed with great success recently by the Engineers on the Ohio and Mississippi Railroad, and which, with the formulas and rules necessary for their application to the laying down of curves, drawn up by Mr. Haslett, one of the Engineers of that Road, are now for the first time given to the public. […] In presenting this work to the public, the Author claims for it the adaptation of a new principle in trigonometrical analysis of the formulas generally used in field calculations. Experience has shown, that versed sines and external secants as frequently enter into calculations on curves as sines and tangents; and by their use, as illustrated in the examples given in this work, it is believed that many of the rules in general use are much simplified, and many calculations concerning curves and running lines made less intricate, and results obtained with more accuracy and far less trouble, than by any methods laid down in works of this kind. The examples given have all been suggested by actual practice, and will explain themselves. […] As a book for practical use in field work, it is confidently believed that this is more direct in the application of rules and facility of calculation than any work now in use. In addition to the tables generally found in books of this kind, the author has prepared, with great labor, a Table of Natural and Logarithmic Versed Sines and External Secants, calculated to degrees, for every minute; also, a Table of Radii and their Logarithms, from 1° to 60°. […]
1856 edition - 1 2 3 4 Allen, Calvin Frank (1894) [1889]. Railroad Curves and Earthwork. New York, USA; London, UK: Spon & Chamberlain; E. & F. Spon, Ltd. Retrieved 2015-11-16.
- 1 2 Nagle, James C. (1897). "IV.138.-165.: Transition Curves; Table XIII.: Natural Versines and Exsecants". Field Manual for Railroad Engineers (1 ed.). New York, USA: John Wiley and Sons, Chapman and Hall, Limited. pp. 110–142, 332–354. Retrieved 2015-11-16.
- 1 2 "Field Manual for Railroad Engineers" (PDF). The Engineer (Review): 540. 1897-12-03. Archived (PDF) from the original on 2015-11-17. Retrieved 2015-11-17.
- 1 2 3 4 5 6 7 8 9 10 11 12 13 Hall, Arthur Graham; Frink, Fred Goodrich (January 1909). "Review Exercises [100] Secondary Trigonometric Functions". Written at Ann Arbor, Michigan, USA. Trigonometry. Vol. Part I: Plane Trigonometry. New York, USA: Henry Holt and Company / Norwood Press / J. S. Cushing Co. - Berwick & Smith Co., Norwood, Massachusetts, USA. p. 125. Retrieved 2017-08-12.
- ↑ Boyer, Carl Benjamin (1969) [1959]. "5: Commentary on the Paper of E. J. Dijksterhuis (The Origins of Classical Mechanics from Aristotle to Newton)". In Clagett, Marshall (ed.). Critical Problems in the History of Science (3 ed.). Madison, Milwaukee, and London: University of Wisconsin Press, Ltd. pp. 185–190. ISBN 0-299-01874-1. LCCN 59-5304. 9780299018740. Retrieved 2015-11-16.
- ↑ Zucker, Ruth (1983) [June 1964]. "Chapter 4.3.147: Elementary Transcendental Functions - Circular functions". In Abramowitz, Milton; Stegun, Irene Ann (eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables. Applied Mathematics Series. Vol. 55 (Ninth reprint with additional corrections of tenth original printing with corrections (December 1972); first ed.). Washington D.C.; New York: United States Department of Commerce, National Bureau of Standards; Dover Publications. p. 78. ISBN 978-0-486-61272-0. LCCN 64-60036. MR 0167642. LCCN 65-12253.
- 1 2 3 4 Calvert, James B. (2007-09-14) [2004-01-10]. "Trigonometry". Archived from the original on 2007-10-02. Retrieved 2015-11-08.
- ↑ Tapson, Frank (2004). "Background Notes on Measures: Angles". 1.4. Cleave Books. Archived from the original on 2007-02-09. Retrieved 2015-11-12.
- 1 2 Patu, Andræâ-Claudio (André Claude); Le Tort, Bartholomæus (February 1745). Rivard, Franciscus (Dominique-François) [in French] (ed.). Theses Mathematicæ De Mathesi Generatim (in Latin). Collegio Dormano–Bellovaco (Collège de Dormans–Beauvais), Paris: Ph. N. Lottin. p. 6. Retrieved 2017-08-06.
- 1 2 Lemonnier, Petro (Pierre) (1750). Genneau, Ludovicum (Ludovico); Rollin, Jacobum (Jacques) (eds.). Physica generalis (in Latin). Vol. 3. Collegio Harcuriano (Collège d'Harcourt), Paris. pp. 303–. Retrieved 2017-08-06.
{{cite book}}:|work=ignored (help) - 1 2 Thysbaert, Jan-Frans (1774). "Articulus II: De situ lineæ rectæ ad Circularem; & de mensura angulorum, quorum vertex non est in circuli centro. §1. De situ lineæ rectæ ad Circularem. Definitio II: [102]". Geometria elementaria et practica (in Latin). Lovanii, e typographia academica. p. 30, foldout. Retrieved 2017-08-06.
- 1 2 van Haecht, Joannes (1784). "Articulus III: De secantibus circuli: Corollarium III: [109]". Geometria elementaria et practica: quam in usum auditorum (in Latin). Lovanii, e typographia academica. p. 24, foldout. Retrieved 2017-08-06.
- 1 2 3 Searles, William Henry (1880-03-01). Field Engineering - A Hand-book of the Theory and Practice of Railway Surveying, Location, and Construction, designed for the Class-room, Field and Office, and containing a large number of useful tables, original and selected (PDF). New York, USA: John Wiley & Sons. Archived (PDF) from the original on 2017-08-13. Retrieved 2017-08-13. 8th revised edition, 1887 16th edition, 1910
- ↑ Cajori, Florian (1952) [March 1929]. A History of Mathematical Notations. Vol. 2 (2 (3rd corrected printing of 1929 issue) ed.). Chicago, USA: Open court publishing company. p. 173. ISBN 978-1-60206-714-1. 1602067147. Retrieved 2015-11-11. (NB. ISBN and link for reprint of 2nd edition by Cosimo, Inc., New York, USA, 2013.)
- ↑ Swanson, Todd; Andersen, Janet; Keeley, Robert (1999). "5 (Trigonometric Functions)" (PDF). Precalculus: A Study of Functions and Their Applications. Harcourt Brace & Company. p. 344. Archived from the original (PDF) on 2003-06-17. Retrieved 2015-11-12.
- 1 2 3 4 Gottschalk, Walter Helbig (2002). Some Quaint & Curious & Almost Forgotten Trig Functions (PDF). Vol. 80. Providence, Rhode Island, USA: Infinite Vistas Press. PVD RI, GG80. Archived (PDF) from the original on 2013-09-25. Retrieved 2015-11-17.
{{cite book}}:|work=ignored (help) - 1 2 3 Frye, Albert I. (1918) [1913]. Civil engineer's pocket-book: a reference-book for engineers, contractors and students containing rules, data, methods, formulas and tables (2 (corrected) ed.). New York, USA; London, UK: D. Van Nostrand Company; Constable and Company, Ltd. Retrieved 2015-11-16.
- ↑ Kenyon, Alfred Monroe; Ingold, Louis (1913). Trigonometry. New York, USA: The Macmillan Company. p. 5. Retrieved 2015-12-08.
- ↑ Hudson, Ralph Gorton; Lipka, Joseph (1917). A Manual of Mathematics. New York, USA: John Wiley & Sons. p. 68. Retrieved 2015-12-08.
- 1 2 3 4 Weisstein, Eric Wolfgang (2015) [2005]. "Exsecant". MathWorld. Wolfram Research, Inc. Archived from the original on 2005-11-29. Retrieved 2015-11-05.
- 1 2 Shaneyfelt, Ted V. "德博士的 Notes About Circles, ज्य, & कोज्य: What in the world is a hacovercosine?". Hilo, Hawaii: University of Hawaii. Archived from the original on 2015-09-19. Retrieved 2015-11-08.
- 1 2 3 Oldham, Keith B.; Myland, Jan C.; Spanier, Jerome (2009) [1987]. "33.13. The Secant sec(x) and Cosecant csc(x) functions - Cognate functions". An Atlas of Functions: with Equator, the Atlas Function Calculator (2 ed.). Springer Science+Business Media, LLC. p. 336. doi:10.1007/978-0-387-48807-3. ISBN 978-0-387-48806-6. LCCN 2008937525.
- 1 2 3 Weisstein, Eric Wolfgang (2015) [2013]. "Excosecant". MathWorld. Wolfram Research, Inc. Archived from the original on 2014-03-26. Retrieved 2015-11-05.
- 1 2 3 Bohannan, Rosser Daniel (1904) [1903]. "$131. The Versed Sine, Exsecant and Coexsecant. §132. Exercises". Plane Trigonometry. Ohio State University: Allyn and Bacon, Boston, USA / J. S. Cushing & Co. — Berwick & Smith Co., Norwood, MA. pp. 235–236. Retrieved 2017-07-09.
- 1 2 3 4 5 6 van Vlijmen, Oscar (2005-12-28) [2003]. "Goniology". Eenheden, constanten en conversies. Archived from the original on 2009-10-28. Retrieved 2015-11-28.
- ↑ Galilei, Galileo (1632). Dialogo di Galileo Galilei sopra i due massimi sistemi del mondo Tolemaico e Copernicano [Dialogue on the Two Chief World Systems, Ptolemaic and Copernican] (in Italian).
- ↑ Galilei, Galileo (1997-05-25) [1632]. Finocchiaro, Maurice A. (ed.). Galileo on the World Systems: A New Abridged Translation and Guide. University of California Press. pp. 184 (n130), 184 (n135), 192 (n158). ISBN 9780520918221. Retrieved 2017-07-30.
[…] Galileo's word is segante (meaning secant), but he clearly intends exsecant; an exsecant is defined as the part of a secant external to the circle […]
- 1 2 Hawking, Stephen William, ed. (2002). On the Shoulders of Giants: The Great Works of Physics and Astronomy. Philadelphia, USA: Running Press. ISBN 0-7624-1698-X. LCCN 2002100441. Retrieved 2017-07-31.
- 1 2 3 Stávek, Jiří (2017-03-10) [2017-02-26]. "On the Hidden Beauty of Trigonometric Functions". Applied Physics Research. Prague, CZ: Canadian Center of Science and Education. 9 (2): 57–64. doi:10.5539/apr.v9n2p57. ISSN 1916-9639. ISSN 1916-9647.
- ↑ Poor, Henry Varnum, ed. (1856-03-22). "PRACTICAL BOOK OF REFERENCE, and Engineer's Field Book. By Charles Haslett, C.E. Edited by Professor Charles W. Hackley, 1 vol. 12mo. Pp. 617. Prico $2.50. Columbia College, N. Y. Stringer & Townsend" (PDF). American Railroad Journal - Steam Navigation, Commerce, Mining, Manufacturers (Review). Second Quarto Series. J. H. Schultz & Co. XII (12): 184. Whole No. 1040, Vol. XX. Retrieved 2017-08-14.
- 1 2 Simpson, David G. (2001-11-08). "AUXTRIG" (Fortran 90 source code). Greenbelt, Maryland, USA: NASA Goddard Space Flight Center. Archived from the original on 2008-06-16. Retrieved 2015-10-26.
- 1 2 van den Doel, Kees (2010-01-25). "jass.utils Class Fmath". JASS - Java Audio Synthesis System. 1.25. Archived from the original on 2007-09-02. Retrieved 2015-10-26.