吉布斯现象
吉布斯现象(英語:),由亨利·威爾布里厄姆于1848年最先提出[1],并由约西亚·吉布斯于1899年证明[2]。在工程应用时常用有限正弦项正弦波叠加逼近原周期信号。所用的谐波次数N的大小决定逼近原波形的程度,N增加,逼近的精度不断改善。但是由于对于具有不连续点的周期信号会发生一种现象:当选取的傅里叶级数的项数N增加时,合成的波形虽然更逼近原函数,但在不连续点附近会出现一个固定高度的过冲,N越大,过冲的最大值越靠近不连续点,但其峰值并不下降,而是大约等于原函数在不连续点处跳变值的9%,且在不连续点两侧呈现衰减振荡的形式。
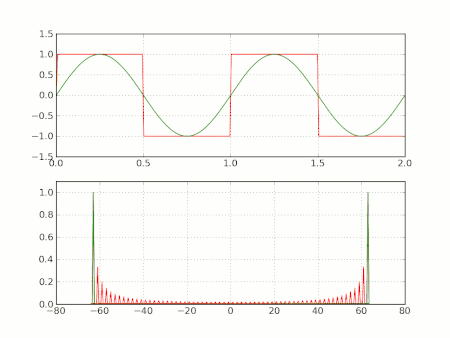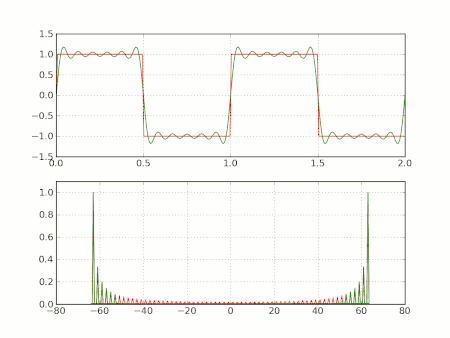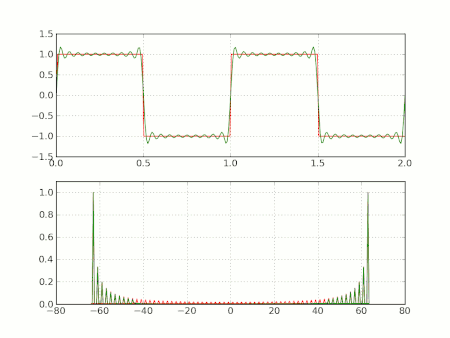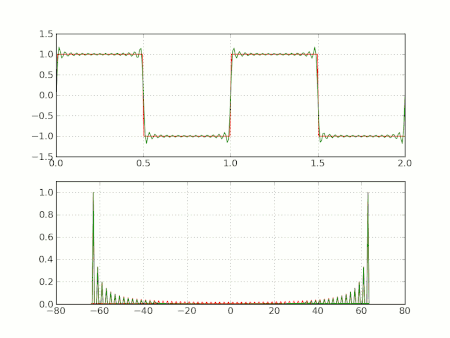
方波動畫示例。當頻率提升時,吉布斯现象尤其明顯

約西亞·吉布斯
参考文献
- Hewitt, Edwin; Hewitt, Robert E. . Archive for History of Exact Sciences. 1979, 21 (2): 129–160 [16 September 2011]. doi:10.1007/BF00330404. Available on-line at: National Chiao Tung University: Open Course Ware: Hewitt & Hewitt, 1979.
- Andrew Dimarogonas. . ISBN 0-13-462938-8.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.