幾何變換
幾何變換(geometric transformation)是指從具有幾何結構之集合至其自身或其他此類集合的一種對射。具體來說,「幾何變換是一個函數,其定義域與值域為點集合。幾何變換最常見的定義域與值域為同時為R2,或同時為R3。其他的幾何變換則要求須為一對一函數,使之有反函數[1]。」可透過研究這些變換的方法來研究幾何[2]。
幾何變換可以其操作集合的維度來分類(因此可分類出平面變換與空間變換等)。幾何變換亦可依據其保留其性質來分類:
以上每種變換均包含前一種變換[4]。
以法國地圖為例:
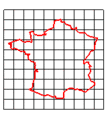 原圖案
原圖案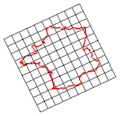
.gif) 仿射變換
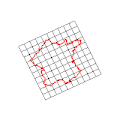
仿射變換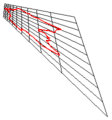 投影變換
投影變換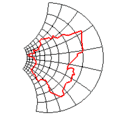
- 共形變換保留角度在一階的相似。
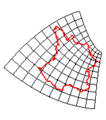 共形變換
共形變換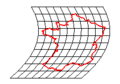 保積變換
保積變換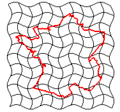

相同類型的群變換可能是其他變換群的子群。
另見
- 愛爾蘭根綱領
- 拓撲學
- 剛性變換
參考資料
- Zalman Usiskin, Anthony L. Peressini, Elena Marchisotto – Mathematics for High School Teachers: An Advanced Perspective, page 84.
- Venema, Gerard A., , Pearson Prentice Hall: 285, 2006, ISBN 9780131437005
- 幾何變換,第131頁,載於Google圖書
- Leland Wilkinson, D. Wills, D. Rope, A. Norton, R. Dubbs – 幾何變換,第182頁,載於Google圖書
- stevecheng. (PDF). planetmath.org. 2013-03-13 [2014-10-01]. (原始内容存档 (PDF)于2014-07-14).
- 幾何變換,第191頁,載於Google圖書 Bruce E. Meserve – Fundamental Concepts of Geometry, page 191.]
| 维基共享资源中相关的多媒体资源:幾何變換 |
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.