正多边形镶嵌

全用同一种正多边形来铺满整塊平面，叫正镶嵌图。正镶嵌图有3种，也只有這3種。只有正三角形、正方形、正六邊形才能如此镶嵌。

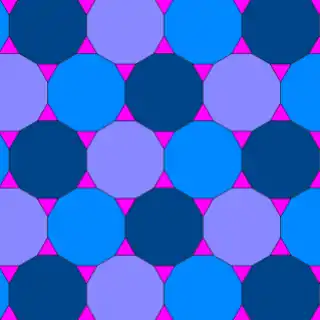
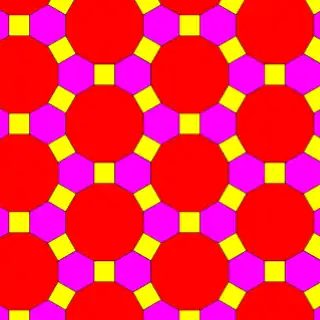
用不同正多边形铺满整塊平面，但交叉点周围的正多边形种类和顺序都相同叫半正镶嵌图。半正镶嵌图有8种。

• Grünbaum, Branko; Shephard, G. C.. . W. H. Freeman and Company. 1987. ISBN 978-0-7167-1193-3.
• D. Chavey. . Computers & Mathematics with Applications. 1989, 17: 147–165.

Euclidean and general tiling links:

• Dutch, Steve. . [2006-09-09]. （原始内容存档于2006年9月9日）.

• Mitchell, K. . [2006-09-09]. （原始内容存档于2021-02-23）.

• Weisstein, Eric W. . MathWorld–A Wolfram Web Resource. [2006-09-09]. （原始内容存档于2021-04-02）.

Hyperbolic tiling links:

• Eppstein, David. . [2006-09-09]. （原始内容存档于2021-01-05）.

• Hatch, Don. . [2006-09-09]. （原始内容存档于2006-09-28）.

• Joyce, David. . [2006-09-09]. （原始内容存档于2006-09-10）.