牛頓-寇次公式

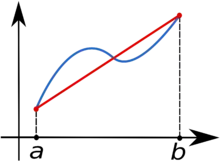
原函數（藍色）近似為紅色的線性函數

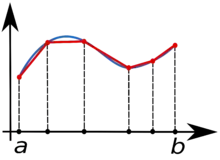
多重梯形法則

梯形法則是：

${\displaystyle \int _{a}^{b}f(x)\,dx\approx (b-a){\frac {f(a)+f(b)}{2}}.}$

這等同將被積函數近似為直線函數，被積的部分近似為梯形。

要求得較準確的數值，可以將要求積的區間分成多個小區間，再個別估計，即：

${\displaystyle \int _{a}^{b}f(x)\,dx\approx {\frac {b-a}{n}}\left({f(a)+f(b) \over 2}+\sum _{k=1}^{n-1}f\left(a+k{\frac {b-a}{n}}\right)\right).}$

可改寫成

${\displaystyle \int _{a}^{b}f(x)\,dx\approx {\frac {b-a}{2n}}\left(f(x_{0})+2f(x_{1})+2f(x_{2})+\cdots +2f(x_{n-1})+f(x_{n})\right)}$

其中

對${\displaystyle k=0,1,\dots ,n}$，${\displaystyle x_{k}=a+k{\frac {b-a}{n}},}$。

辛普森法则（Simpson's rule，又稱森遜法則）是：

${\displaystyle \int _{a}^{b}f(x)\,dx\approx {\frac {b-a}{6}}\left[f(a)+4f\left({\frac {a+b}{2}}\right)+f(b)\right].}$

同樣地，辛普森法则也有多重的版本：

${\displaystyle \int _{a}^{b}f(x)\,dx\approx {\frac {h}{3}}\cdot \left[f(x_{0})+2\sum _{k=1}^{n-1}f(x_{k})+4\sum _{k=1}^{n}f\left({\frac {x_{k-1}+x_{k}}{2}}\right)+f(x_{n})\right]}$

${\displaystyle h={\frac {b-a}{n}},\ x_{k}=a+k\cdot h.}$

或寫成

${\displaystyle \int _{a}^{b}f(x)\,dx\approx {\frac {h}{3}}{\bigg [}f(x_{0})+4f(x_{1})+2f(x_{2})+4f(x_{3})+2f(x_{4})+\cdots +4f(x_{n-1})+f(x_{n}){\bigg ]}}$

牛頓-寇次公式（Newton-Cotes rule / Newton-Cotes formula）以Roger Cotes和艾薩克·牛頓命名。其內容是：

${\displaystyle \int _{a}^{b}f(x)\,dx\approx \sum _{i=0}^{n}w_{i}\,f(x_{i})}$

其中對${\displaystyle k=0,1,\dots ,n}$，${\displaystyle w_{i}}$是常數（由${\displaystyle n}$的值決定），${\displaystyle x_{k}=a+k{\frac {b-a}{n}}}$。

梯形法則和辛卜生法則便是${\displaystyle n=1,2}$的情況。

亦有不採用在邊界點來估計的版本，即取 ${\displaystyle x_{k}=a+k{\frac {b-a}{n+1}}}$ 。

例子

下表中${\displaystyle f_{i}=f(x_{i})}$，${\displaystyle \xi \in [a,b]}$，${\displaystyle h=b-a}$

精度	名稱	公式	誤差
1	梯形法則	${\displaystyle {\frac {h}{2}}(f_{0}+f_{1})}$	${\displaystyle -{\frac {2h^{3}}{3}}\,f^{(2)}(\xi )}$
2	辛卜生法則	${\displaystyle {\frac {h}{6}}(f_{0}+4f_{1}+f_{2})}$	${\displaystyle -{\frac {h^{5}}{90}}\,f^{(4)}(\xi )}$
3	辛卜生3/8法則 辛卜生第二法則	${\displaystyle {\frac {h}{8}}(f_{0}+3f_{1}+3f_{2}+f_{3})}$	${\displaystyle -{\frac {3h^{5}}{80}}\,f^{(4)}(\xi )}$
4	保爾法則 （Boole's rule ／ Bode's rule）	${\displaystyle {\frac {2h}{45}}(7f_{0}+32f_{1}+12f_{2}+32f_{3}+7f_{4})}$	${\displaystyle -{\frac {8h^{7}}{945}}\,f^{(6)}(\xi )}$
不用界點的
0	中點法	${\displaystyle 2hf_{1}\,}$	${\displaystyle {\frac {h^{3}}{24}}\,f^{(2)}(\xi )}$
1		${\displaystyle {\frac {3h}{2}}(f_{1}+f_{2})}$	${\displaystyle {\frac {h^{3}}{4}}\,f^{(2)}(\xi )}$
2		${\displaystyle {\frac {4h}{3}}(2f_{1}-f_{2}+2f_{3})}$	${\displaystyle {\frac {28h^{5}}{90}}f^{(4)}(\xi )}$
3		${\displaystyle {\frac {5h}{24}}(11f_{1}+f_{2}+f_{3}+11f_{4})}$	${\displaystyle {\frac {95h^{5}}{144}}f^{(4)}(\xi )}$

• M. Abramowitz and I. A. Stegun, eds. Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables. New York: Dover, 1972. (See Section 25.4.)
• George E. Forsythe, Michael A. Malcolm, and Cleve B. Moler. Computer Methods for Mathematical Computations. Englewood Cliffs, NJ: Prentice-Hall, 1977. (See Section 5.1.)
• William H. Press, Brian P. Flannery, Saul A. Teukolsky, William T. Vetterling. Numerical Recipes in C. Cambridge, UK: Cambridge University Press, 1988. (See Section 4.1.)
• Josef Stoer and Roland Bulirsch. Introduction to Numerical Analysis. New York: Springer-Verlag, 1980. (See Section 3.1.)

• 黃 Sir 的計算機網頁: Numerical Integration : Trapezoidal Rule and Simpson's Rule （页面存档备份，存于）