莱斯分布
在概率论與数理統計领域,萊斯分布(Rice distribution或Rician distribution)是一種连续概率分布,以美国科学家斯蒂芬·莱斯的名字命名,其概率密度函数为:
|
密度函數  Rice probability density functions for various v with σ=1. 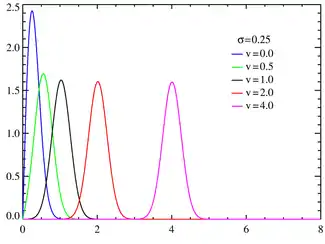 Rice probability density functions for various v with σ=0.25. | |||
|
累積分布函數 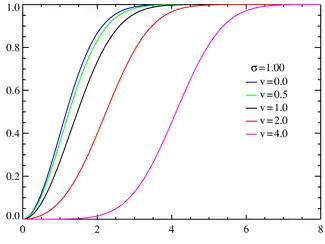 Rice cumulative density functions for various v with σ=1. 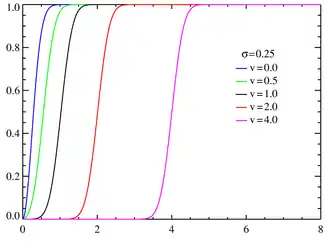 Rice cumulative density functions for various v with σ=0.25. | |||
|
| |||
| 值域 | |||
| 期望值 | |||
| 偏度 | (complicated) | ||
| 峰度 | (complicated) | ||
极限情况
For large values of the argument, the Laguerre polynomial becomes (See Abramowitz and Stegun §13.5.1 (页面存档备份,存于))
It is seen that as becomes large or becomes small the mean becomes and the variance becomes
相關條目
- Stephen O. Rice (1907-1986)
- 瑞利分布
- 莱斯衰落
外部連結
- Yongjun Xie and Yuguang Fang, "A General Statistical Channel Model for Mobile Satellite Systems" IEEE Transactions on Vehicular Technology, VOL. 49, NO. 3, MAY 2000. http://www.fang.ece.ufl.edu/mypaper/tvt00_xie.pdf (页面存档备份,存于)
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.