證明黎曼ζ函數的歐拉乘積公式

黎曼ζ函數以歐拉乘積的方式可寫成

${\displaystyle \sum _{n=1}^{\infty }{\frac {1}{n^{s}}}=\prod _{p{\text{ prime}}}{\frac {1}{1-p^{-s}}}}$

而左方等於黎曼ζ函數：

${\displaystyle \zeta (s)=\sum _{n=1}^{\infty }{\frac {1}{n^{s}}}=1+{\frac {1}{2^{s}}}+{\frac {1}{3^{s}}}+{\frac {1}{4^{s}}}+{\frac {1}{5^{s}}}+\ldots }$

右方的乘積則擴展至所有質數p：

${\displaystyle \prod _{p{\text{ prime}}}{\frac {1}{1-p^{-s}}}={\frac {1}{1-2^{-s}}}\cdot {\frac {1}{1-3^{-s}}}\cdot {\frac {1}{1-5^{-s}}}\cdot {\frac {1}{1-7^{-s}}}\cdots {\frac {1}{1-p^{-s}}}\cdots }$

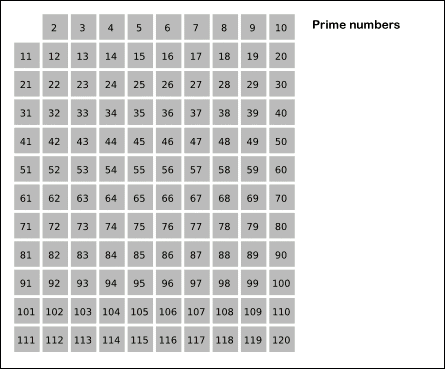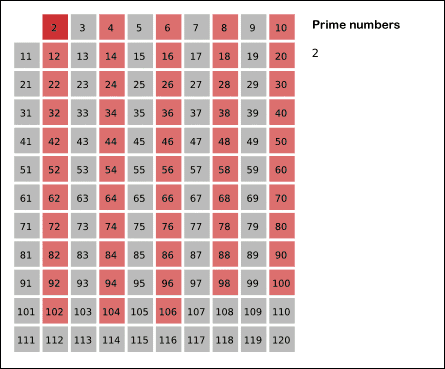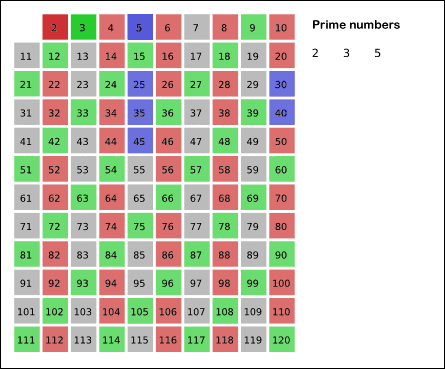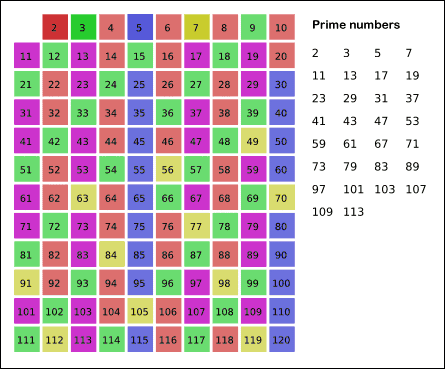
證明方法採用了埃拉托斯特尼篩法的概念，此篩法用於找尋出特定範圍內的質數。

證明過程只需用到簡單的代數概念，這亦是歐拉當初使用的證明方法。

${\displaystyle \zeta (s)=1+{\frac {1}{2^{s}}}+{\frac {1}{3^{s}}}+{\frac {1}{4^{s}}}+{\frac {1}{5^{s}}}+\ldots }$（1）

${\displaystyle {\frac {1}{2^{s}}}\zeta (s)={\frac {1}{2^{s}}}+{\frac {1}{4^{s}}}+{\frac {1}{6^{s}}}+{\frac {1}{8^{s}}}+{\frac {1}{10^{s}}}+\ldots }$（2）

從（1）式減去（2）式：

${\displaystyle \left(1-{\frac {1}{2^{s}}}\right)\zeta (s)=1+{\frac {1}{3^{s}}}+{\frac {1}{5^{s}}}+{\frac {1}{7^{s}}}+{\frac {1}{9^{s}}}+{\frac {1}{11^{s}}}+{\frac {1}{13^{s}}}+\ldots }$（3）

重複上面步驟：

${\displaystyle {\frac {1}{3^{s}}}\left(1-{\frac {1}{2^{s}}}\right)\zeta (s)={\frac {1}{3^{s}}}+{\frac {1}{9^{s}}}+{\frac {1}{15^{s}}}+{\frac {1}{21^{s}}}+{\frac {1}{27^{s}}}+{\frac {1}{33^{s}}}+\ldots }$（4）

從（3）式減去（4）式，可得：

${\displaystyle \left(1-{\frac {1}{3^{s}}}\right)\left(1-{\frac {1}{2^{s}}}\right)\zeta (s)=1+{\frac {1}{5^{s}}}+{\frac {1}{7^{s}}}+{\frac {1}{11^{s}}}+{\frac {1}{13^{s}}}+{\frac {1}{17^{s}}}+\ldots }$

這次2和3的所有倍數項都被減去。可見右方的的倍數項可被篩去，不斷重複以上步驟可得：

${\displaystyle \ldots \left(1-{\frac {1}{11^{s}}}\right)\left(1-{\frac {1}{7^{s}}}\right)\left(1-{\frac {1}{5^{s}}}\right)\left(1-{\frac {1}{3^{s}}}\right)\left(1-{\frac {1}{2^{s}}}\right)\zeta (s)=1}$

左右兩方除以所有括號項，我們得到：

${\displaystyle \zeta (s)={\frac {1}{\left(1-{\frac {1}{2^{s}}}\right)\left(1-{\frac {1}{3^{s}}}\right)\left(1-{\frac {1}{5^{s}}}\right)\left(1-{\frac {1}{7^{s}}}\right)\left(1-{\frac {1}{11^{s}}}\right)\ldots }}}$

最後，公式可寫成質數的無窮乘積：

${\displaystyle \zeta (s)=\prod _{p{\text{ prime}}}{\frac {1}{1-p^{-s}}}}$

證畢。

為了使證明更嚴密，我們只需注意到當${\displaystyle \Re (s)>1}$，已篩的右方項趨向1，並遵從狄利克雷級數的收歛性。

從以上公式可推導出 ζ(1) 的有趣結果。

${\displaystyle \ldots \left(1-{\frac {1}{11}}\right)\left(1-{\frac {1}{7}}\right)\left(1-{\frac {1}{5}}\right)\left(1-{\frac {1}{3}}\right)\left(1-{\frac {1}{2}}\right)\zeta (1)=1}$

可以寫成，

${\displaystyle \ldots \left({\frac {10}{11}}\right)\left({\frac {6}{7}}\right)\left({\frac {4}{5}}\right)\left({\frac {2}{3}}\right)\left({\frac {1}{2}}\right)\zeta (1)=1}$

${\displaystyle \left({\frac {\ldots \cdot 10\cdot 6\cdot 4\cdot 2\cdot 1}{\ldots \cdot 11\cdot 7\cdot 5\cdot 3\cdot 2}}\right)\zeta (1)=1}$

又知：

${\displaystyle \zeta (1)=1+{\frac {1}{2}}+{\frac {1}{3}}+{\frac {1}{4}}+{\frac {1}{5}}+\ldots }$

所以

${\displaystyle 1+{\frac {1}{2}}+{\frac {1}{3}}+{\frac {1}{4}}+{\frac {1}{5}}+\ldots ={\frac {2\cdot 3\cdot 5\cdot 7\cdot 11\cdot \ldots }{1\cdot 2\cdot 4\cdot 6\cdot 10\cdot \ldots }}}$

我們得知左式是調和級數，並發散至無窮大，故此右式的分子（質數階乘）必定同樣發散至無窮大。由此可以證明質數有無限多個。

• 黎曼ζ函數
• 歐拉乘積
• 狄利克雷級數
• 埃拉托斯特尼篩法
• 質數

• John Derbyshire, Prime Obsession: Bernhard Riemann and The Greatest Unsolved Problem in Mathematics, Joseph Henry Press, 2003, ISBN 978-0-309-08549-6