闭运算

在數學形態學中，集合B對集合A的Closing是先dilation再用erosion。

A\bullet B=(A\oplus B)\ominus B,\,

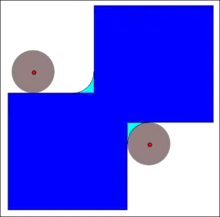
如果選擇灰色圓盤當 B(kernel)，對兩個深藍色的正方形做closing，會多出淺藍色的部分.

$\oplus$ 和 $\ominus$ 分别表示dilation和erosion。

在影像處理中，closing和opening是用來對影像除噪，這邊通常A就是我們輸入的影像，B則是kernel。

特性

Idempotent，即 $(A\bullet B)\bullet B=A\bullet B$ .
Increasing，如果 $A\subseteq C$ ，然後 $A\bullet B\subseteq C\bullet B$ .
Extensive，即 $A\subseteq A\bullet B$ .
Translation invariant.

實作

這邊對binary image做closing的操作，kernel為5*5大小，4個角落被去掉。

closing不一定要先dilation一次再用erosion一次，也可以使用closing = dilation k times + erosion k times。

當想要closing的洞比較大時，可以使用更高的k次。

def dilation(img):
  img1 = np.zeros((img.shape[0], img.shape[1]), dtype="int")
  for i in range(img.shape[0]):
    for j in range(img.shape[1]):
      if img[i][j]>0:
        row = i-2; col = j-2; # The octogonal 4-5-5-5-4 kernel
        for w in range(5):
          for h in range(5):
            if ( w==0 and h==0 ) or ( w==0 and h==4 ) or ( w==4 and h==0 ) or ( w==4 and h==4 ):
              continue
            else:
              i2 = row + w; j2 = col + h;
              if i2 >= 0 and j2 >= 0 and i2 < img.shape[0] and j2 < img.shape[1]:
                img1[i2][j2] = 255
  return img1

def erosion(img):
  img1 = np.zeros((img.shape[0], img.shape[1]), dtype="int")
  for i in range(img.shape[0]):
    for j in range(img.shape[1]):
        flag = 0
        row = i-2; col = j-2; # The octogonal 4-5-5-5-4 kernel
        for w in range(5):
          for h in range(5):
            if ( w==0 and h==0 ) or ( w==0 and h==4 ) or ( w==4 and h==0 ) or ( w==4 and h==4 ):
              continue
            else:
              i2 = row + w; j2 = col + h;
              if i2 > 0 and j2 > 0 and i2 < img.shape[0] and j2 < img.shape[1]:
                if img[i2][j2] == 0:
                  flag = 1
        if flag == 0:
          img1[i][j] = 255
  return img1
  
def closing(img):
  return erosion(dilation(img))

參考

Image Analysis and Mathematical Morphology by Jean Serra, ISBN 0-12-637240-3 (1982)
Image Analysis and Mathematical Morphology, Volume 2: Theoretical Advances by Jean Serra, ISBN 0-12-637241-1 (1988)
An Introduction to Morphological Image Processing by Edward R. Dougherty, ISBN 0-8194-0845-X (1992)

This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.