Coshc函数
Coshc函数常见于有关光学散射[1]、海森堡时空[2]和双曲几何学的论文中[3]其定义如下:[4][5]
它是下列微分方程的一个解:
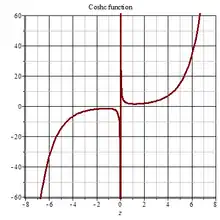
Coshc 2D plot
_2D_plot.png.webp)
Coshc'(z) 2D plot
- 复域虚部
- 复域实部
- 绝对值
- 一阶导数
- 导数实部
- 导数虚部
- 导数绝对值
表示为其他特殊函数
级数展开
图集
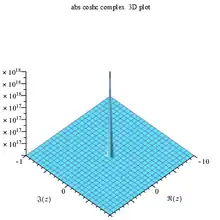 Coshc abs complex 3D |
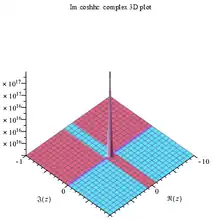 Coshc Im complex 3D plot |
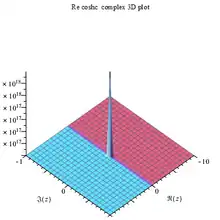 Coshc Re complex 3D plot |
_Im_complex_3D_plot.png.webp) Coshc'(z) Im complex 3D plot |
_Re_complex_3D_plot.png.webp) Coshc'(z) Re complex 3D plot |
_abs_complex_3D_plot.png.webp) Coshc'(z) abs complex 3D plot |
_abs_density_plot.JPG.webp) Coshc'(x) abs density plot |
_Im_density_plot.JPG.webp) Coshc'(x) Im density plot |
_Re_density_plot.JPG.webp) Coshc'(x) Re density plot |
参考文献
- PN Den Outer, TM Nieuwenhuizen, A Lagendijk,Location of objects in multiple-scattering media,JOSA A, Vol. 10, Issue 6, pp. 1209-1218 (1993)
- T Körpinar ,New characterizations for minimizing energy of biharmonic particles in Heisenberg spacetime - International Journal of Theoretical Physics, 2014 - Springer
- Nilg¨un S¨onmez,A Trigonometric Proof of the Euler Theorem in Hyperbolic Geometry,International Mathematical Forum, 4, 2009, no. 38, 1877 - 1881
- JHM ten Thije Boonkkamp, J van Dijk, L Liu,Extension of the complete flux scheme to systems of conservation laws,J Sci Comput (2012) 53:552–568,DOI 10.1007/s10915-012-9588-5
- Weisstein, Eric W. "Coshc Function." From MathWorld--A Wolfram Web Resource. http://mathworld.wolfram.com/CoshcFunction.html%5B%5D
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.