
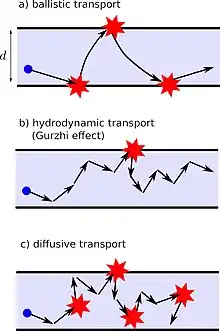
The Gurzhi effect was theoretically predicted[1][2] by Radii Gurzhi in 1963, and it consists of decreasing of electric resistance of a finite size conductor with increasing of its temperature (i.e. the situation for some temperature interval). Gurzhi effect usually being considered as the evidence of electron hydrodynamic transport[3][4][5][6][7][8] in conducting media. The mechanism of Gurzhi effect is the following. The value of the resistance of the conductor is inverse to the — a mean free path corresponding to the momentum loss from the electrons+phonons system
where is the average distance which electron pass between two consequence interactions with a boundary, and is a mean free path corresponding to other possibilities of momentum loss. The electron reflection from the boundary is assumed to be diffusive.
When temperature is low we have ballistic transport with , , where is a width of the conductor, is a mean free path corresponding to effective normal electron-electron collisions (i.e. collisions without total electrons+phonons momentum loss). For low temperatures phonon emitted by electron quickly interacts with another electron without loss of total electron+phonons momentum and , where is a mean free path corresponding to the electron-phonon collisions. Also we assume . Thus the resistance for lowest temperatures is a constant (see the picture). The Gurzhi effect appears when the temperature is increased to have . In this regime the electron diffusive length between two consequence interaction with the boundary can be considered as momentum loss free path: , and the resistance is proportional to , and thus we have a negative derivative . Therefore, Gurzhi effect can be observed when .
Gurzhi effect corresponds to unusual situation when electrical resistance depends on a frequency of normal collisions. As one can see this effect appears due to the presence of a boundaries with finite characteristic size . Later Gurzhi's group discovered a special role of electron hydrodynamics in a spin transport.[9][10] In such a case magnetic inhomogeneity plays role of a "boundary" with spin-diffusion length[11] as a characteristic size instead of as before. This magnetic inhomogeneity stops electrons of the one spin component which becomes an effective scatterers for electrons of another spin component. In this case magnetoresistance of a conductor depends on the frequency of normal electron-electron collisions as well as in the Gurzhi effect.
References
- ↑ Gurzhi, R. N. (1963). "Minimum of resistance in impurity-free conductors". J Exp Theor Phys. 17: 521.
- ↑ Gurzhi, R. N. (1968). "HYDRODYNAMIC EFFECTS IN SOLIDS AT LOW TEMPERATURE". Soviet Physics Uspekhi. 11 (2): 255–270. doi:10.1070/PU1968v011n02ABEH003815.
- ↑ Yu, Z. -Z.; Haerle, M.; Zwart, J. W.; Bass, J.; Pratt, W. P.; Schroeder, P. A. (1984). "Negative Temperature Derivative of Resistivity in Thin Potassium Samples: The Gurzhi Effect?". Phys. Rev. Lett. 52 (5): 368–371. doi:10.1103/PhysRevLett.52.368.
- ↑ de Jong, M. J. M.; Molenkamp, L. W. (1995). "Hydrodynamic electron flow in high-mobility wires". Phys. Rev. B. 51 (19): 13389–13402. arXiv:cond-mat/9411067. doi:10.1103/PhysRevB.51.13389.
- ↑ Alekseev, P. S. (2016). "Negative Magnetoresistance in Viscous Flow of Two-Dimensional Electrons". Phys. Rev. Lett. 117 (16): 166601. arXiv:1603.04587. doi:10.1103/PhysRevLett.117.166601.
- ↑ Narozhny, Boris N.; Gornyi, Igor V.; Mirlin, Alexander D.; Schmalian, Jörg (2017). "Hydrodynamic Approach to Electronic Transport in Graphene". Annalen der Physik. 529 (11): 1700043. arXiv:1704.03494. doi:10.1002/andp.201700043.
- ↑ Moll, Philip J. W.; Kushwaha, Pallavi; Nandi, Nabhanila; Schmidt, Burkhard; Mackenzie, Andrew P. (2016). "Evidence for hydrodynamic electron flow in PdCoO2". Science. 351 (6277): 1061–1064. doi:10.1126/science.aac8385. hdl:10023/8262.
- ↑ Scaffidi, Thomas; Nandi, Nabhanila; Schmidt, Burkhard; Mackenzie, Andrew P.; Moore, Joel E. (2017). "Hydrodynamic Electron Flow and Hall Viscosity". Phys. Rev. Lett. 118 (22): 226601. doi:10.1103/PhysRevLett.118.226601. hdl:10023/11223.
- ↑ Gurzhi, R. N.; Kalinenko, A. N.; Kopeliovich, A. I.; Pyshkin, P. V.; Yanovsky, A. V. (2006). "Dynamics of a spin-polarized electron liquid: Spin oscillations with a low decay". Phys. Rev. B. 73 (15): 153204. arXiv:1109.1872. doi:10.1103/PhysRevB.73.153204.
- ↑ Gurzhi, R. N.; Kalinenko, A. N.; Kopeliovich, A. I.; Pyshkin, P. V.; Yanovsky, A. V. (2011). "Electrical resistance of spatially varying magnetic interfaces. The role of normal scattering". Low Temperature Physics. 37 (2): 149–156. arXiv:1109.0555. doi:10.1063/1.3556662.
- ↑ Bass, J.; Pratt, W. P. (2007). "Spin-diffusion lengths in metals and alloys, and spin-flipping at metal/metal interfaces: an experimentalist's critical review". Journal of Physics: Condensed Matter. 19: 183201. arXiv:cond-mat/0610085. doi:10.1088/0953-8984/19/18/183201.