Kirnberger temperament is an irregular temperament developed in the second half of the 18th century by Johann Kirnberger. Kirnberger was a student of Johann Sebastian Bach, held great admiration for his teacher and was one of his principal proponents.[1]
The first Kirnberger temperament, "Kirnberger I", had similarities to Pythagorean temperament, which stressed the importance of perfect fifths all throughout the circle of fifths. A complete circle of perfect fifths is not possible, because when the circle comes to an end at the tone it began, it will have overshot its original pitch. Thus, if one tunes C–G, G–D, D–A, A–E, E–B, B–F♯, F♯–C♯, C♯–G♯ (A♭), A♭–E♭, E♭–B♭, B♭–F, F–C … the new "C" will not be the same frequency as the first. The two "C"s will have a discrepancy of about 23 cents (a comma), which would be unacceptable. This difference between the initial "c" and final "c" that is derived from performing a series of perfect tunings is generally referred to as the Pythagorean comma. In Kirnberger I, the D–A fifth is reduced by a syntonic comma, making the major thirds F–A, C–E, G–B, and D–F# pure, though the fifth based on D is a ratio of 40/27 instead of 3/2. Many tuning systems have been developed to "spread around" that comma, that is, to divide that anomalous musical space among the other intervals of the scale.
Practical Temperaments: Kirnberger II
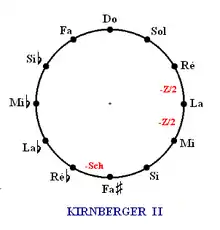
Kirnberger's first method of compensating for and closing the circle of fifths was to split the "wolf" interval, known to those who have used meantone temperaments, in half between two different fifths. That is, to compensate for the one extra comma, he removed half a comma from two of the formerly perfect fifths in order to complete the circle. In so doing, he allowed the remaining fifths to stay pure. At the time, however, pure thirds were more valued than pure fifths (meantone temperament had eight pure thirds and sacrificed four entire chords to achieve this end.) So, Kirnberger allowed for three pure thirds, the rest being slightly wide and the worst being three Pythagorean thirds (22 cents wider than pure) on the opposite end of the circle from the pure thirds. To put it graphically:
C-----G-----D------A-----E-----B-----F♯-----C♯-----Ab(G♯)-----Eb-----Bb-----F-----C
p p −½ −½ p p p p p p p p
|__________pure 3rd______|
|__________pure 3rd______|
|_______pure 3rd________|
|__________Pythag. 3rd_________|
|_________Pythag. 3rd___________|
|________Pythag. 3rd___________|
The above table represents Kirnberger II temperament. The first row under the intervals shows either a "p" for pure, or "−½" for those intervals narrowed to close the circle of fifths (D–A), (A–E). Below these are shown the pure 3rds (between C–E, G–B, D–F♯), and Pythagorean (very wide) 3rds (B–D♯, F♯–A♯(B♭), D♭–F.)
Temperament, however, is a give-and-take situation: None is a perfect solution. It must also be remembered that temperament applies only to instruments with fixed pitch: Any keyboard instrument, lutes, viols, and so forth. Wind musicians, singers, and string players all have a degree of control over the exact pitch and intonation of what they play, and may therefore be free of such restrictive systems. When the two classes of players come together, it is important when evaluating a temperament to consider the tendencies of the instruments vs. those of the temperament. Kirnberger II would only have been applicable to harps and keyboard instruments such as the harpsichord and organ. The advantages of this system are its three pure major thirds and its ten pure fifths; the disadvantages are, of course, the two narrow "half-wolf" fifths and the three Pythagorean, super-wide thirds. The chords are not entirely unusable but certainly must not be used frequently nor in close succession within the course of a piece.
Kirnberger III

After some disappointment with his sour, narrow fifths, Kirnberger experimented further and developed another possibility, later named the Kirnberger III.
This temperament splits the Syntonic comma between four fifths instead of two; ¼-comma tempered fifths are used extensively in meantone and are much easier to tune and to listen to. This also eliminates two of the three pure thirds found in Kirnberger II. Therefore, only one third remains pure (between C and E), and there are fewer Pythagorean thirds. A greater middle ground is reached in this improvement, and each key is closer to being equal to the next. The drawback is an aesthetic one: Fewer chords have pure thirds and fifths. But every temperament system is a mix of give-and-take compromises; each finds a way of dealing with the comma.
See also
Further reading
- Klop, G.C. (1974). Harpsichord Tuning. Raleigh, NC: The Sunbury Press. — called "Kirnberger 2" in Lieberman & Miller (2006). Lou Harrison. p. 80. ISBN 0-252-03120-2; presumably other Kirnberger temperaments as well.
- Kroesbergen, Willem; Cruickshank, Andrew (2014). 18th century quotes on J.S. Bach's temperament (Report) – via academia.edu.
- Eckersley, Dominic (April 2013). Rosetta revisited (PDF) (Report) – via wordpress.com.