The molecular clock is a figurative term for a technique that uses the mutation rate of biomolecules to deduce the time in prehistory when two or more life forms diverged. The biomolecular data used for such calculations are usually nucleotide sequences for DNA, RNA, or amino acid sequences for proteins. The benchmarks for determining the mutation rate are often fossil or archaeological dates. The molecular clock was first tested in 1962 on the hemoglobin protein variants of various animals, and is commonly used in molecular evolution to estimate times of speciation or radiation. It is sometimes called a gene clock or an evolutionary clock.

Early discovery and genetic equidistance

The notion of the existence of a so-called "molecular clock" was first attributed to Émile Zuckerkandl and Linus Pauling who, in 1962, noticed that the number of amino acid differences in hemoglobin between different lineages changes roughly linearly with time, as estimated from fossil evidence.[1] They generalized this observation to assert that the rate of evolutionary change of any specified protein was approximately constant over time and over different lineages (known as the molecular clock hypothesis).

The genetic equidistance phenomenon was first noted in 1963 by Emanuel Margoliash, who wrote: "It appears that the number of residue differences between cytochrome c of any two species is mostly conditioned by the time elapsed since the lines of evolution leading to these two species originally diverged. If this is correct, the cytochrome c of all mammals should be equally different from the cytochrome c of all birds. Since fish diverges from the main stem of vertebrate evolution earlier than either birds or mammals, the cytochrome c of both mammals and birds should be equally different from the cytochrome c of fish. Similarly, all vertebrate cytochrome c should be equally different from the yeast protein."[2] For example, the difference between the cytochrome c of a carp and a frog, turtle, chicken, rabbit, and horse is a very constant 13% to 14%. Similarly, the difference between the cytochrome c of a bacterium and yeast, wheat, moth, tuna, pigeon, and horse ranges from 64% to 69%. Together with the work of Emile Zuckerkandl and Linus Pauling, the genetic equidistance result led directly to the formal postulation of the molecular clock hypothesis in the early 1960s.[3]

Similarly, Vincent Sarich and Allan Wilson in 1967 demonstrated that molecular differences among modern Primates in albumin proteins showed that approximately constant rates of change had occurred in all the lineages they assessed.[4] The basic logic of their analysis involved recognizing that if one species lineage had evolved more quickly than a sister species lineage since their common ancestor, then the molecular differences between an outgroup (more distantly related) species and the faster-evolving species should be larger (since more molecular changes would have accumulated on that lineage) than the molecular differences between the outgroup species and the slower-evolving species. This method is known as the relative rate test. Sarich and Wilson's paper reported, for example, that human (Homo sapiens) and chimpanzee (Pan troglodytes) albumin immunological cross-reactions suggested they were about equally different from Ceboidea (New World Monkey) species (within experimental error). This meant that they had both accumulated approximately equal changes in albumin since their shared common ancestor. This pattern was also found for all the primate comparisons they tested. When calibrated with the few well-documented fossil branch points (such as no Primate fossils of modern aspect found before the K-T boundary), this led Sarich and Wilson to argue that the human-chimp divergence probably occurred only ~4–6 million years ago.[5]

Relationship with neutral theory

The observation of a clock-like rate of molecular change was originally purely phenomenological. Later, the work of Motoo Kimura[6] developed the neutral theory of molecular evolution, which predicted a molecular clock. Let there be N individuals, and to keep this calculation simple, let the individuals be haploid (i.e. have one copy of each gene). Let the rate of neutral mutations (i.e. mutations with no effect on fitness) in a new individual be . The probability that this new mutation will become fixed in the population is then 1/N, since each copy of the gene is as good as any other. Every generation, each individual can have new mutations, so there are N new neutral mutations in the population as a whole. That means that each generation, new neutral mutations will become fixed. If most changes seen during molecular evolution are neutral, then fixations in a population will accumulate at a clock-rate that is equal to the rate of neutral mutations in an individual.

Calibration

To use molecular clocks to estimate divergence times, molecular clocks need to be "calibrated". This is because molecular data alone does not contain any information on absolute times. For viral phylogenetics and ancient DNA studies—two areas of evolutionary biology where it is possible to sample sequences over an evolutionary timescale—the dates of the intermediate samples can be used to calibrate the molecular clock. However, most phylogenies require that the molecular clock be calibrated using independent evidence about dates, such as the fossil record.[7] There are two general methods for calibrating the molecular clock using fossils: node calibration and tip calibration.[8]

Node calibration

Sometimes referred to as node dating, node calibration is a method for time-scaling phylogenetic trees by specifying time constraints for one or more nodes in the tree. Early methods of clock calibration only used a single fossil constraint (e.g. non-parametric rate smoothing),[9] but newer methods (BEAST[10] and r8s[11]) allow for the use of multiple fossils to calibrate molecular clocks. The oldest fossil of a clade is used to constrain the minimum possible age for the node representing the most recent common ancestor of the clade. However, due to incomplete fossil preservation and other factors, clades are typically older than their oldest fossils.[8] In order to account for this, nodes are allowed to be older than the minimum constraint in node calibration analyses. However, determining how much older the node is allowed to be is challenging. There are a number of strategies for deriving the maximum bound for the age of a clade including those based on birth-death models, fossil stratigraphic distribution analyses, or taphonomic controls.[12] Alternatively, instead of a maximum and a minimum, a probability density can be used to represent the uncertainty about the age of the clade. These calibration densities can take the shape of standard probability densities (e.g. normal, lognormal, exponential, gamma) that can be used to express the uncertainty associated with divergence time estimates. [10] Determining the shape and parameters of the probability distribution is not trivial, but there are methods that use not only the oldest fossil but a larger sample of the fossil record of clades to estimate calibration densities empirically.[13] Studies have shown that increasing the number of fossil constraints increases the accuracy of divergence time estimation.[14]

Tip calibration

Sometimes referred to as tip dating, tip calibration is a method of molecular clock calibration in which fossils are treated as taxa and placed on the tips of the tree. This is achieved by creating a matrix that includes a molecular dataset for the extant taxa along with a morphological dataset for both the extinct and the extant taxa.[12] Unlike node calibration, this method reconstructs the tree topology and places the fossils simultaneously. Molecular and morphological models work together simultaneously, allowing morphology to inform the placement of fossils.[8] Tip calibration makes use of all relevant fossil taxa during clock calibration, rather than relying on only the oldest fossil of each clade. This method does not rely on the interpretation of negative evidence to infer maximum clade ages.[12]

Expansion calibration

Demographic changes in populations can be detected as fluctuations in historical coalescent effective population size from a sample of extant genetic variation in the population using coalescent theory.[15][16][17] Ancient population expansions that are well documented and dated in the geological record can be used to calibrate a rate of molecular evolution in a manner similar to node calibration. However, instead of calibrating from the known age of a node, expansion calibration uses a two-epoch model of constant population size followed by population growth, with the time of transition between epochs being the parameter of interest for calibration.[18][19] Expansion calibration works at shorter, intraspecific timescales in comparison to node calibration, because expansions can only be detected after the most recent common ancestor of the species in question. Expansion dating has been used to show that molecular clock rates can be inflated at short timescales[18] (< 1 MY) due to incomplete fixation of alleles, as discussed below[20][21]

Total evidence dating

This approach to tip calibration goes a step further by simultaneously estimating fossil placement, topology, and the evolutionary timescale. In this method, the age of a fossil can inform its phylogenetic position in addition to morphology. By allowing all aspects of tree reconstruction to occur simultaneously, the risk of biased results is decreased.[8] This approach has been improved upon by pairing it with different models. One current method of molecular clock calibration is total evidence dating paired with the fossilized birth-death (FBD) model and a model of morphological evolution.[22] The FBD model is novel in that it allows for "sampled ancestors", which are fossil taxa that are the direct ancestor of a living taxon or lineage. This allows fossils to be placed on a branch above an extant organism, rather than being confined to the tips.[23]

Methods

Bayesian methods can provide more appropriate estimates of divergence times, especially if large datasets—such as those yielded by phylogenomics—are employed.[24]

Non-constant rate of molecular clock

Sometimes only a single divergence date can be estimated from fossils, with all other dates inferred from that. Other sets of species have abundant fossils available, allowing the hypothesis of constant divergence rates to be tested. DNA sequences experiencing low levels of negative selection showed divergence rates of 0.7–0.8% per Myr in bacteria, mammals, invertebrates, and plants.[25] In the same study, genomic regions experiencing very high negative or purifying selection (encoding rRNA) were considerably slower (1% per 50 Myr).

In addition to such variation in rate with genomic position, since the early 1990s variation among taxa has proven fertile ground for research too,[26] even over comparatively short periods of evolutionary time (for example mockingbirds[27]). Tube-nosed seabirds have molecular clocks that on average run at half speed of many other birds,[28] possibly due to long generation times, and many turtles have a molecular clock running at one-eighth the speed it does in small mammals, or even slower.[29] Effects of small population size are also likely to confound molecular clock analyses. Researchers such as Francisco J. Ayala have more fundamentally challenged the molecular clock hypothesis.[30][31][32] According to Ayala's 1999 study, five factors combine to limit the application of molecular clock models:

  • Changing generation times (If the rate of new mutations depends at least partly on the number of generations rather than the number of years)
  • Population size (Genetic drift is stronger in small populations, and so more mutations are effectively neutral)
  • Species-specific differences (due to differing metabolism, ecology, evolutionary history, ...)
  • Change in function of the protein studied (can be avoided in closely related species by utilizing non-coding DNA sequences or emphasizing silent mutations)
  • Changes in the intensity of natural selection.
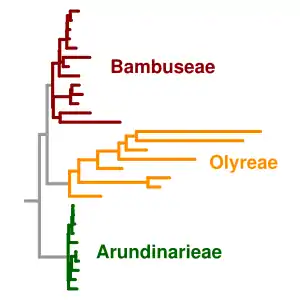
Phylogram showing three groups, one of which has strikingly longer branches than the two others
Woody bamboos (tribes Arundinarieae and Bambuseae) have long generation times and lower mutation rates, as expressed by short branches in the phylogenetic tree, than the fast-evolving herbaceous bamboos (Olyreae).

Molecular clock users have developed workaround solutions using a number of statistical approaches including maximum likelihood techniques and later Bayesian modeling. In particular, models that take into account rate variation across lineages have been proposed in order to obtain better estimates of divergence times. These models are called relaxed molecular clocks[33] because they represent an intermediate position between the 'strict' molecular clock hypothesis and Joseph Felsenstein's many-rates model[34] and are made possible through MCMC techniques that explore a weighted range of tree topologies and simultaneously estimate parameters of the chosen substitution model. It must be remembered that divergence dates inferred using a molecular clock are based on statistical inference and not on direct evidence.

The molecular clock runs into particular challenges at very short and very long timescales. At long timescales, the problem is saturation. When enough time has passed, many sites have undergone more than one change, but it is impossible to detect more than one. This means that the observed number of changes is no longer linear with time, but instead flattens out. Even at intermediate genetic distances, with phylogenetic data still sufficient to estimate topology, signal for the overall scale of the tree can be weak under complex likelihood models, leading to highly uncertain molecular clock estimates.[35]

At very short time scales, many differences between samples do not represent fixation of different sequences in the different populations. Instead, they represent alternative alleles that were both present as part of a polymorphism in the common ancestor. The inclusion of differences that have not yet become fixed leads to a potentially dramatic inflation of the apparent rate of the molecular clock at very short timescales.[21][36]

Uses

The molecular clock technique is an important tool in molecular systematics, macroevolution, and phylogenetic comparative methods. Estimation of the dates of phylogenetic events, including those not documented by fossils, such as the divergences between living taxa has allowed the study of macroevolutionary processes in organisms that had limited fossil records. Phylogenetic comparative methods rely heavily on calibrated phylogenies.

See also

References

  1. ↑ Zuckerkandl E, Pauling (1962). "Molecular disease, evolution, and genic heterogeneity". In Kasha, M., Pullman, B (eds.). Horizons in Biochemistry. Academic Press, New York. pp. 189–225.
  2. ↑ Margoliash E (October 1963). "Primary Structure and Evolution of Cytochrome C". Proceedings of the National Academy of Sciences of the United States of America. 50 (4): 672–679. Bibcode:1963PNAS...50..672M. doi:10.1073/pnas.50.4.672. PMC 221244. PMID 14077496.
  3. ↑ Kumar S (August 2005). "Molecular clocks: four decades of evolution". Nature Reviews. Genetics. 6 (8): 654–662. doi:10.1038/nrg1659. PMID 16136655. S2CID 14261833.
  4. ↑ Sarich VM, Wilson AC (July 1967). "Rates of albumin evolution in primates". Proceedings of the National Academy of Sciences of the United States of America. 58 (1): 142–148. Bibcode:1967PNAS...58..142S. doi:10.1073/pnas.58.1.142. PMC 335609. PMID 4962458.
  5. ↑ Sarich VM, Wilson AC (December 1967). "Immunological time scale for hominid evolution". Science. 158 (3805): 1200–1203. Bibcode:1967Sci...158.1200S. doi:10.1126/science.158.3805.1200. JSTOR 1722843. PMID 4964406. S2CID 7349579.
  6. ↑ Kimura M (February 1968). "Evolutionary rate at the molecular level". Nature. 217 (5129): 624–626. Bibcode:1968Natur.217..624K. doi:10.1038/217624a0. PMID 5637732. S2CID 4161261.
  7. ↑ Benton MJ, Donoghue PC (January 2007). "Paleontological evidence to date the tree of life". Molecular Biology and Evolution. 24 (1): 26–53. doi:10.1093/molbev/msl150. PMID 17047029.
  8. 1 2 3 4 Donoghue PC, Yang Z (July 2016). "The evolution of methods for establishing evolutionary timescales". Philosophical Transactions of the Royal Society of London. Series B, Biological Sciences. 371 (1699): 20160020. doi:10.1098/rstb.2016.0020. PMC 4920342. PMID 27325838.
  9. ↑ Sanderson M (1997). "A nonparametric approach to estimating divergence times in the absence of rate constancy". Molecular Biology and Evolution. 14 (12): 1218–1231. doi:10.1093/oxfordjournals.molbev.a025731. S2CID 17647010.
  10. 1 2 Drummond AJ, Suchard MA, Xie D, Rambaut A (August 2012). "Bayesian phylogenetics with BEAUti and the BEAST 1.7". Molecular Biology and Evolution. 29 (8): 1969–1973. doi:10.1093/molbev/mss075. PMC 3408070. PMID 22367748.
  11. ↑ Sanderson MJ (January 2003). "r8s: inferring absolute rates of molecular evolution and divergence times in the absence of a molecular clock". Bioinformatics. 19 (2): 301–302. doi:10.1093/bioinformatics/19.2.301. PMID 12538260.
  12. 1 2 3 O'Reilly JE, Dos Reis M, Donoghue PC (November 2015). "Dating Tips for Divergence-Time Estimation". Trends in Genetics. 31 (11): 637–650. doi:10.1016/j.tig.2015.08.001. hdl:1983/ba7bbcf4-1d51-4b74-a800-9948edb3bbe6. PMID 26439502.
  13. ↑ Claramunt, S (2022). "CladeDate : Calibration information generator for divergence time estimation". Methods in Ecology and Evolution. Wiley. 13 (11): 2331–2338. doi:10.1111/2041-210x.13977. ISSN 2041-210X. S2CID 252353611.
  14. ↑ Zheng Y, Wiens JJ (April 2015). "Do missing data influence the accuracy of divergence-time estimation with BEAST?". Molecular Phylogenetics and Evolution. 85 (1): 41–49. doi:10.1016/j.ympev.2015.02.002. PMID 25681677. S2CID 3895351.
  15. ↑ Rogers AR, Harpending H (May 1992). "Population growth makes waves in the distribution of pairwise genetic differences". Molecular Biology and Evolution. 9 (3): 552–569. doi:10.1093/oxfordjournals.molbev.a040727. PMID 1316531.
  16. ↑ Shapiro B, Drummond AJ, Rambaut A, Wilson MC, Matheus PE, Sher AV, et al. (November 2004). "Rise and fall of the Beringian steppe bison". Science. 306 (5701): 1561–1565. Bibcode:2004Sci...306.1561S. doi:10.1126/science.1101074. PMID 15567864. S2CID 27134675.
  17. ↑ Li H, Durbin R (July 2011). "Inference of human population history from individual whole-genome sequences". Nature. 475 (7357): 493–496. doi:10.1038/nature10231. PMC 3154645. PMID 21753753.
  18. 1 2 Crandall ED, Sbrocco EJ, Deboer TS, Barber PH, Carpenter KE (February 2012). "Expansion dating: calibrating molecular clocks in marine species from expansions onto the Sunda Shelf Following the Last Glacial Maximum". Molecular Biology and Evolution. 29 (2): 707–719. doi:10.1093/molbev/msr227. PMID 21926069.
  19. ↑ Hoareau TB (May 2016). "Late Glacial Demographic Expansion Motivates a Clock Overhaul for Population Genetics". Systematic Biology. 65 (3): 449–464. doi:10.1093/sysbio/syv120. hdl:2263/53371. PMID 26683588.
  20. ↑ Ho SY, Tong KJ, Foster CS, Ritchie AM, Lo N, Crisp MD (September 2015). "Biogeographic calibrations for the molecular clock". Biology Letters. 11 (9): 20150194. doi:10.1098/rsbl.2015.0194. PMC 4614420. PMID 26333662.
  21. 1 2 Ho SY, Phillips MJ, Cooper A, Drummond AJ (July 2005). "Time dependency of molecular rate estimates and systematic overestimation of recent divergence times". Molecular Biology and Evolution. 22 (7): 1561–1568. doi:10.1093/molbev/msi145. PMID 15814826.
  22. ↑ Heath TA, Huelsenbeck JP, Stadler T (July 2014). "The fossilized birth-death process for coherent calibration of divergence-time estimates". Proceedings of the National Academy of Sciences of the United States of America. 111 (29): E2957–E2966. arXiv:1310.2968. Bibcode:2014PNAS..111E2957H. doi:10.1073/pnas.1319091111. PMC 4115571. PMID 25009181.
  23. ↑ Gavryushkina A, Heath TA, Ksepka DT, Stadler T, Welch D, Drummond AJ (January 2017). "Bayesian Total-Evidence Dating Reveals the Recent Crown Radiation of Penguins". Systematic Biology. 66 (1): 57–73. arXiv:1506.04797. doi:10.1093/sysbio/syw060. PMC 5410945. PMID 28173531.
  24. ↑ dos Reis M, Inoue J, Hasegawa M, Asher RJ, Donoghue PC, Yang Z (September 2012). "Phylogenomic datasets provide both precision and accuracy in estimating the timescale of placental mammal phylogeny". Proceedings. Biological Sciences. 279 (1742): 3491–3500. doi:10.1098/rspb.2012.0683. PMC 3396900. PMID 22628470.
  25. ↑ Ochman H, Wilson AC (1987). "Evolution in bacteria: evidence for a universal substitution rate in cellular genomes". Journal of Molecular Evolution. 26 (1–2): 74–86. Bibcode:1987JMolE..26...74O. doi:10.1007/BF02111283. PMID 3125340. S2CID 8260277.
  26. ↑ Douzery EJ, Delsuc F, Stanhope MJ, Huchon D (2003). "Local molecular clocks in three nuclear genes: divergence times for rodents and other mammals and incompatibility among fossil calibrations". Journal of Molecular Evolution. 57 (Suppl 1): S201–S213. Bibcode:2003JMolE..57S.201D. CiteSeerX 10.1.1.535.897. doi:10.1007/s00239-003-0028-x. PMID 15008417. S2CID 23887665.
  27. ↑ Hunt JS, Bermingham E, Ricklefs RE (2001). "Molecular systematics and biogeography of Antillean thrashers, tremblers, and mockingbirds (Aves: Mimidae)". Auk. 118 (1): 35–55. doi:10.1642/0004-8038(2001)118[0035:MSABOA]2.0.CO;2. ISSN 0004-8038. S2CID 51797284.
  28. ↑ Rheindt, F. E. & Austin, J. (2005). "Major analytical and conceptual shortcomings in a recent taxonomic revision of the Procellariiformes – A reply to Penhallurick and Wink (2004)" (PDF). Emu. 105 (2): 181–186. doi:10.1071/MU04039. S2CID 20390465.
  29. ↑ Avise JC, Bowen BW, Lamb T, Meylan AB, Bermingham E (May 1992). "Mitochondrial DNA evolution at a turtle's pace: evidence for low genetic variability and reduced microevolutionary rate in the Testudines". Molecular Biology and Evolution. 9 (3): 457–473. doi:10.1093/oxfordjournals.molbev.a040735. PMID 1584014.
  30. ↑ Ayala FJ (January 1999). "Molecular clock mirages". BioEssays. 21 (1): 71–75. doi:10.1002/(SICI)1521-1878(199901)21:1<71::AID-BIES9>3.0.CO;2-B. PMID 10070256. Archived from the original on 16 December 2012.
  31. ↑ Schwartz, J. H. & Maresca, B. (2006). "Do Molecular Clocks Run at All? A Critique of Molecular Systematics". Biological Theory. 1 (4): 357–371. CiteSeerX 10.1.1.534.4502. doi:10.1162/biot.2006.1.4.357. S2CID 28166727.
  32. ↑ Pascual-García A, Arenas M, Bastolla U (November 2019). "The Molecular Clock in the Evolution of Protein Structures". Systematic Biology. 68 (6): 987–1002. doi:10.1093/sysbio/syz022. hdl:20.500.11850/373053. PMID 31111152.
  33. ↑ Drummond AJ, Ho SY, Phillips MJ, Rambaut A (May 2006). "Relaxed phylogenetics and dating with confidence". PLOS Biology. 4 (5): e88. doi:10.1371/journal.pbio.0040088. PMC 1395354. PMID 16683862.
  34. ↑ Felsenstein J (2001). "Taking variation of evolutionary rates between sites into account in inferring phylogenies". Journal of Molecular Evolution. 53 (4–5): 447–455. Bibcode:2001JMolE..53..447F. doi:10.1007/s002390010234. PMID 11675604. S2CID 9791493.
  35. ↑ Marshall, D. C., et al. 2016. Inflation of molecular clock rates and dates: molecular phylogenetics, biogeography, and diversification of a global cicada radiation from Australasia (Hemiptera: Cicadidae: Cicadettini). Systematic Biology 65(1):16–34.
  36. ↑ Peterson GI, Masel J (November 2009). "Quantitative prediction of molecular clock and ka/ks at short timescales". Molecular Biology and Evolution. 26 (11): 2595–2603. doi:10.1093/molbev/msp175. PMC 2912466. PMID 19661199.

Further reading

This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.