正三角形鑲嵌

在幾何學中，正三角形鑲嵌、又稱為正三角方格[3]是一種正多邊形在平面上的密鋪，又稱正鑲嵌圖。

命名

康威稱正三角形鑲嵌為deltille。deltille一詞來自於外形為三角形的希臘字母 Delta （Δ），有時也稱作六角化正六邊形鑲嵌。

由於正三角形鑲嵌是由正三角形組成，又因正三角形內角為60度，因此每個頂點周圍都有6個三角形，且剛好占滿360度。

正三角形鑲嵌在施萊夫利符號中，用{3,6}表示。

正三角形鑲嵌是三個的平面正鑲嵌圖之一。另外兩個是正方形鑲嵌和正六邊形鑲嵌。

一般將畫在紙上的正三角方格稱作正三角格紙[3]，正三角格紙是用來畫三維立體圖或三維透視圖用的。使用正三角格紙作圖會比較容易做出三維立體圖或三維透視圖，而且圖形看起來比較接近三維[3]。

正三角形鑲嵌有九種不同的上色方式，他們依頂點周為顏色數來命名： 111111, 111112, 111212, 111213, 111222, 112122, 121212, 121213, 121314。

正三角形镶嵌的顶点排布被称作A₂晶格[4]。正三角形镶嵌是单纯形堆砌家族的二维成员。

A₂^*晶格（又称A₂³），可由所有3种A₂晶格组合得来，就等价于A₂晶格。

+

=

的对偶 =

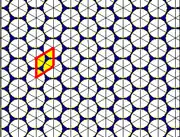
以正三角形镶嵌的顶点为圆心，我们可以得到二维的最密圆堆砌，每个圆都与6个相邻圆接触（接触数），堆砌密度为 ${\frac {\pi }{\sqrt {12}}}$ 或90.69%。由于3个A₂晶格组合还是A₂晶格，这种圆堆砌种的圆可被涂成三种颜色。

A₂晶格的沃罗诺伊图是正六边形镶嵌，它也是正三角形镶嵌的对偶。因此，正六边形镶嵌也与最密圆堆砌有直接的对应关系。

Coxeter, H.S.M. Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8 p. 296, Table II: Regular honeycombs
Grünbaum, Branko ; and Shephard, G. C. . New York: W. H. Freeman. 1987. ISBN 0-7167-1193-1. (Chapter 2.1: Regular and uniform tilings, p. 58-65)
埃里克·韦斯坦因. . MathWorld.
- 埃里克·韦斯坦因. . MathWorld.
- 埃里克·韦斯坦因. . MathWorld.
Klitzing, Richard. . bendwavy.org.
Williams, Robert. . Dover Publications, Inc. 1979. ISBN 0-486-23729-X. p35
John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5
Tilings and Patterns, from list of 107 isohedral tilings, p.473-481

This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.

	球面鑲嵌	多面體	歐式鑲嵌	緊湊雙曲鑲嵌				仿緊空間	非緊空間
n	1	2	3	4	5	6		∞
2n邊形鑲嵌	{2,3}	{4,3}	{6,3}	{8,3}	{10,3}	{12,3}		{∞,3}	{iπ/λ,3}
交錯2n邊形鑲嵌	h{2,3}	h{4,3}	h{6,3}	h{8,3}	h{10,3}	h{12,3}	...	h{∞,3}	h{iπ/λ,3}