四階八邊形鑲嵌

該鑲嵌有四種均勻構造，其中三種是透過從[8,8]萬花筒中移除鏡射線而形成的。 在二階以及四階頂點間移除鏡射線 [8,8,1⁺]，會成為[(8,8,4)], (*884)對稱性。 在[8,4^*]中移除兩條鏡射線，剩餘的鏡射線則為*4444對稱性。

四階八邊形鑲嵌的四種均勻塗色

均勻塗色
對稱性	[8,4] (*842)	[8,8] (*882) =	[(8,4,8)] = [8,8,1+] (*884) = =	[1+,8,8,1+] (*4444) =
圖示	{8,4}	r{8,8}	r(8,4,8) = r{8,8}1⁄2	r{8,4}1⁄8 = r{8,8}1⁄4
考斯特圖			= =	= = =

可表示以正六邊形的八邊鏡射的雙曲萬花筒。 這種由八個二階交叉反射的對稱性在軌形符號被稱為(*22222222)或著(*2⁸)。 在考斯特表示法可表示為[8^*,4]， 從三個的鏡射線當中移除兩條穿過八邊形中心的鏡射線。 在原本六邊形基礎中對所有的兩個頂點加入中垂線則可以限定出一個偏方面體44222對稱群；加入對角線則可以限定出一個*444對稱群；加入中垂線則可以限定出一個*4222對稱群；全部加入則限定出了一個*842對稱群。

*444

*4222

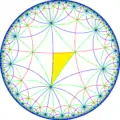*832

該鑲嵌有一種表面塗色，即將八邊形交錯塗上不同顏色。該表面塗色的圖形可以用t₁{8,8}的施萊夫利符號表示，是一種半正鑲嵌，稱為截半八階八邊形鑲嵌

該鑲嵌在拓撲學中和每個面皆為八邊形的多面體及鑲嵌相關， 從正八邊形鑲嵌，施萊夫利符號皆為{8,n}，而考斯特符號為，從n到無窮。

球面	雙曲鑲嵌
8.8	83	84	85	86	87	88	...8∞

該鑲嵌在拓樸學中也和每個頂點有著四個面的多面體及鑲嵌相關，由正八面體開始， 施萊夫利符號皆為{n,4}，而考斯特符號為，從n到無窮。

球面鑲嵌		多面體	雙曲鑲嵌
24	34	44	54	64	74	84	...∞4

{3,4}

{4,4}

{5,4}

{6,4}

{7,4}

{8,4}

...

{∞,4}

维基共享资源中相关的多媒体资源：四階八邊形鑲嵌

• 正方形鑲嵌
• 正八邊形鑲嵌

• John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 19, The Hyperbolic Archimedean Tessellations)
• . . Dover Publications. 1999. ISBN 0-486-40919-8. LCCN 99035678.

• 埃里克·韦斯坦因. . MathWorld.
• 埃里克·韦斯坦因. . MathWorld.
• Hyperbolic and Spherical Tiling Gallery（页面存档备份，存于）
• KaleidoTile 3: Educational software to create spherical, planar and hyperbolic tilings （页面存档备份，存于）
• Hyperbolic Planar Tessellations, Don Hatch（页面存档备份，存于）