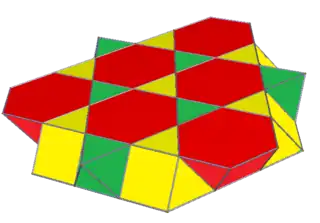
截半立方體堆砌

在幾何學中，截半立方體堆砌是一種歐幾里得三維空間的半正堆砌，由截半立方體和正八面體堆砌而成，是三維空間內28個半正密鋪之一，其對偶多面體為雙四角錐堆砌。

截半立方體堆砌
線架圖
類型均勻堆砌
維度3
對偶多胞形雙四角錐堆砌
類比截半正方形鑲嵌
數學表示法
考克斯特符號
node 4 node_1 3 node 4 node 
node 4 node_1 split1 nodes  = node 4 node_1 3 node 4 node_h0 
node_1 split1 nodes split2 node_1  = node_h0 4 node_1 split1 nodes  = node_h0 4 node_1 3 node 4 node_h0 
纖維流形記號4−:2
施萊夫利符號r{4,3,4} or t1{4,3,4}
r{3[4]}
性質
胞r{4,3}
{3,4}
面{3}
{4}
組成與佈局
顶点图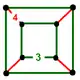
長方體
對稱性
對稱群
空間群Pm3m (221)
考克斯特群, [4,3,4]
特性
顶点正

康威稱截半立方體堆砌為cuboctahedrille[1]，因為它可以藉由立方體堆砌經過「截半」變換構造而來，也可以視為由截半立方體堆砌而得，但截半立方體無法單獨堆砌，必須和其他多面體一起堆砌，而截半立方體堆砌是由截半立方體和正八面體共同堆砌而得。

表面塗色
對稱性 [4,3,4]
 [1+,4,3,4]
[4,31,1], 		[4,3,4,1+]
[4,31,1], 		[1+,4,3,4,1+]
[3[4]],
空間群Pm3m
(221)		Fm3m
(225)		Fm3m
(225)		F43m
(216)
表面塗色
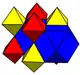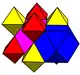考克斯特符號 node 4 node_1 3 node 4 node  node 4 node_1 3 node 4 node_h0  node_h0 4 node_1 3 node 4 node  node_h0 4 node_1 3 node 4 node_h0 
node 4 node_1 split1 nodes  nodes_11 split2 node 4 node  node_1 split1 nodes split2 node_1 
頂點圖
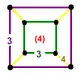頂點
值
對稱性		 D4h
[4,2]
(*224)
order 16		 D2h
[2,2]
(*222)
order 8		 C4v
[4]
(*44)
order 8		 C2v
[2]
(*22)
order 4

結構

截半立方體堆砌可以被切割出一個截半六邊形鑲嵌的面，從截半立方體的六邊形中心切割，創建了兩個正三角帳塔。這部分的結構均勻，可用考克斯特記號node_h 2x node_h 6 node 3 node_1 表示，符號為s3{2,6,3}。

参考文獻
  • George Olshevsky, Uniform Panoploid Tetracombs, Manuscript (2006) （包含11个凸半正镶嵌、28个凸半正堆砌、和143个凸半正四维砌的全表）
  • Kaleidoscopes: Selected Writings of H.S.M. Coxeter, F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication参与编辑, 1995, ISBN 978-0-471-01003-6
    • (22页) H.S.M.考克斯特, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10] (1.9 半正空间镶嵌)
  • A. Andreini, Sulle reti di poliedri regolari e semiregolari e sulle corrispondenti reti correlative (On the regular and semiregular nets of polyhedra and on the corresponding correlative nets), Mem. Società Italiana della Scienze, Ser.3, 14 (1905) 75–129.
  1. John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, (2008) The Symmetries of Things, ISBN 978-1-56881-220-5 (Chapter 21, Naming the Archimedean and Catalan polyhedra and tilings, Architectonic and Catoptric tessellations, p 292-298, includes all the nonprismatic forms)
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.