截角五维超正方体
截角五维超正方体可以通过在每条棱距离顶点处截断五维超正方体的顶点来得到。每个被截断的顶点会产生一个新的正五胞体。
| 截角五维超正方体 | |
|---|---|
 | |
| 類型 | 五维均匀多胞体 |
| 維度 | 5 |
| 數學表示法 | |
| 考克斯特符號 | |
| 施萊夫利符號 | t{4,3,3,3} |
| 性質 | |
| 四维胞 | 42 |
| 胞 | 200 |
| 面 | 400 |
| 邊 | 400 |
| 頂點 | 160 |
| 組成與佈局 | |
| 顶点图 | Elongated tetrahedral pyramid |
| 對稱性 | |
| 考克斯特群 | BC5, [3,3,3,4] |
| 特性 | |
| convex | |
投影
| 考克斯特平面 | B5 | B4 / D5 | B3 / D4 / A2 |
|---|---|---|---|
| Graph |  |
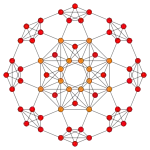 |
 |
| 二面体群 | [10] | [8] | [6] |
| 考克斯特平面 | B2 | A3 | |
| Graph |  |
 | |
| 二面体群 | [4] | [4] |
截角五维超正方体是各维度截角超方形中的第四个:
 |
  |
  |
  |
  |
  |
  |
... |
| 八边形 | 截角立方体 | 截角正八胞体 | 截角五维超正方体 | 截角六维超正方体 | 截角七维超正方体 | 截角八维超正方体 | |
参考文献
- H.S.M. Coxeter:
- H.S.M. Coxeter, Regular Polytopes, 3rd Edition, Dover New York, 1973
- Kaleidoscopes: Selected Writings of H.S.M. Coxeter, editied by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6 (页面存档备份,存于)
- (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10]
- (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II, [Math. Zeit. 188 (1985) 559-591]
- (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
- Norman Johnson Uniform Polytopes, Manuscript (1991)
- N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D.
- Klitzing, Richard. . bendwavy.org. o3o3o3x4x - tan, o3o3x3x4o - bittin
外部链接
- 埃里克·韦斯坦因. . MathWorld.
- Olshevsky, George, Measure polytope at Glossary for Hyperspace.
- Polytopes of Various Dimensions
- Multi-dimensional Glossary (页面存档备份,存于)
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.