多元正态分布
多变量正态分布亦称为多变量高斯分布。它是单维正态分布向多维的推广。它同矩阵正态分布有紧密的联系。
|
密度函數 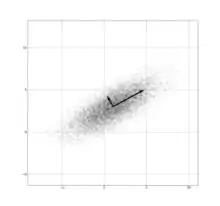 Many samples from a multivariate (bivariate) Gaussian distribution centered at (1,3) with a standard deviation of 3 in roughly the (0.878, 0.478) direction (longer vector) and of 1 in the second direction (shorter vector, orthogonal to the longer vector). | |||
| 记号 | |||
|---|---|---|---|
|
μ ∈ RN — 位置 Σ ∈ RN×N — 协方差矩阵 (半正定) | |||
| 值域 | x ∈ μ+span(Σ) ⊆ RN | ||
|
(仅当 Σ 为正定矩阵时) | |||
| 累積分布函數 | 解析表达式不存在 | ||
| 期望值 | μ | ||
| 眾數 | μ | ||
| Σ | |||
| 熵 | |||
| 特徵函数 | |||
一般形式
N维随机向量如果服从多变量正态分布,必须满足下面的三个等價条件:
如果是非奇异的,那么该分布可以由以下的概率密度函数来描述:[1]
注意这里的表示协方差矩阵的行列式。
- 二元的情况
在二维非奇异的情况下(k = rank(Σ) = 2),向量 [X Y]′ 的概率密度函数为:
其中 ρ 是 X 与 Y 之间的相关系数, 且 。在这种情况下,
参考文献
- UIUC, Lecture 21. The Multivariate Normal Distribution (页面存档备份,存于), 21.5:"Finding the Density".
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.