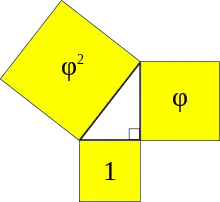
开普勒三角
作开普勒三角形

通过黄金矩形,用尺规作图作开普勒三角
數學巧合

正方形和圓的周長近似相等
若繪製一個三邊為的开普勒三角形,並且考慮
- 其外接圓
- 邊長等於(三角形中數值介於中間的邊長)的正方形
則正方形的周長()和圓的周長()相當接近,誤差小於0.1%。
這是因為的數學巧合,上述的圓和正方形其周長不可能相同,若是相同,就可以求解化圓為方的不可能問題了。換句話說,因為是超越數。
參考資料
- . [2016-12-28]. (原始内容存档于2011-09-02).
- The Great Pyramid, The Great Discovery, and The Great Coincidence, Mark Herkommer, June 24, 2008 (Web archive)
- Markowsky, George. (PDF). College Mathematics Journal (Mathematical Association of America). January 1992, 23 (1): 2–19. JSTOR 2686193. doi:10.2307/2686193. (原始内容存档 (PDF)于2020-12-11).
It does not appear that the Egyptians even knew of the existence of φ much less incorporated it in their buildings
参见
- 黄金菱形
- 黄金三角形
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.