正七邊形鑲嵌
在幾何學中,正七邊形鑲嵌(英語:)是一種由正七邊形拼合,並且將正七邊形重複排列組合,並讓圖形完全拼合,而且沒有空隙或重疊的幾何構造。
 龐加萊圓盤模型 | ||||
| 類別 | 雙曲正鑲嵌 | |||
|---|---|---|---|---|
| 對偶多面體 | 七階三角形鑲嵌 | |||
| 識別 | ||||
| 鮑爾斯縮寫 | heat | |||
| 數學表示法 | ||||
| 考克斯特符號 | ||||
| 施萊夫利符號 | {7,3} | |||
| 威佐夫符號 | 3 | 7 2 | |||
| 組成與佈局 | ||||
| 頂點圖 | 73 | |||
| 對稱性 | ||||
| 對稱群 | [7,3], (*732) | |||
| 圖像 | ||||
| ||||
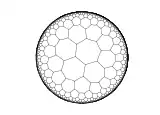
正七邊形鑲嵌是一種雙曲正鑲嵌,由正七邊形組成,在施萊夫利符號中以{7,3}來表示,因為每個頂點周圍都有3個正七邊形。
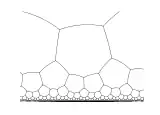
三個正七邊形由於超過360度,因此無法在平面作出,但若硬將正七邊形邊對邊接合,將會變成一個馬鞍形,且每個頂點皆會落在一個雙曲拋物面上。
正七邊形鑲嵌無法在一個平面上構造,因為每個頂點的角度超過了360度,但可以在一個雙曲拋物面上構造[1],因此正七邊形鑲嵌也是羅式幾何或雙曲幾何中討論的幾何構造。
 一個正七邊形鑲嵌的紙模型,可以看到它不是一個平面,像是一個馬鞍面 |
相關半正鑲嵌
正七邊形鑲嵌在拓扑上与一系列用施萊夫利符號{n,3}與{7,n}表示的(广义)多面体一直延伸到双曲镶嵌:
| 多面体 | 欧式镶嵌 | 双曲镶嵌 | ||||||
|---|---|---|---|---|---|---|---|---|
 {2,3} |
 {3,3} |
 {4,3} |
{5,3} |
 {6,3} |
 {7,3} |
 {8,3} |
... |  {∞,3} |
當n大於2時,所有{7,n}都是双曲镶嵌:
 {7,3} |
 {7,4} |
 {7,5} |
 {7,6} |
 {7,7} |
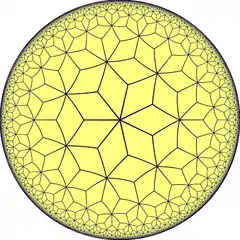
正七邊形鑲嵌可以透過截角操作或其他康威變換得到一系列与之相关的半正镶嵌,其與正七邊形鑲嵌擁有相似的對稱性[7,3](*732)或[7,3]+(732):
| 對稱群:[7,3], (*732) | [7,3]+, (732) | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
 |
 |
 |
 |
 |
 |
 |
 | |||
| {7,3} | t{7,3} | r{7,3} | 2t{7,3}=t{3,7} | 2r{7,3}={3,7} | rr{7,3} | tr{7,3} | sr{7,3} | |||
| 半正對偶 | ||||||||||
 |
 |
 |
 |
 |
 |
 |
 | |||
| V73 | V3.14.14 | V3.7.3.7 | V6.6.7 | V37 | V3.4.7.4 | V4.6.14 | V3.3.3.3.7 | |||
赫爾維茨曲面

產生這種鑲嵌的對稱群(2,3,7)根本域為(2,3,7)施瓦茨三角形的三角群
七階三角形鑲嵌的對稱群是(2,3,7)三角群,且其根本域為(2,3,7)施瓦茨三角形。這是最小的雙曲施瓦茨三角形,因此,由赫爾維茨的同構定理的證明,該鑲嵌完全密鋪整個赫爾維茨曲面(黎曼曲面與最大對稱群),給出一個三角對稱群等於其構群為黎曼曲面。其中最小的赫爾維茨曲面是克萊因商(Klein quartic)虧格3、168階、包含56個七邊形鑲嵌在一起,形成24個頂點。
其對偶七階三角形鑲嵌具有相同的對稱群,因而產生三角形鑲嵌赫爾維曲面。
參考文獻
- Arlan Ramsay, Robert D. Richtmyer, Introduction to Hyperbolic Geometry, Springer; 1 edition (December 16, 1995)
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 19, The Hyperbolic Archimedean Tessellations)
- . . Dover Publications. 1999. ISBN 0-486-40919-8. LCCN 99035678.
- 埃里克·韦斯坦因. . MathWorld.
- 埃里克·韦斯坦因. . MathWorld.
- Hyperbolic and Spherical Tiling Gallery (页面存档备份,存于)
- KaleidoTile 3: Educational software to create spherical, planar and hyperbolic tilings (页面存档备份,存于)
- Hyperbolic Planar Tessellations, Don Hatch (页面存档备份,存于)
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.