均勻多面體
在幾何學中,均勻多面體是指由正多邊形面構成且具有頂點可遞特性的多面體,點可遞代表該幾何結構中的任2個頂點其中一個頂點可以透過平移、旋轉與鏡射的過程映射到另一個頂點,換句話說這個幾何結構的頂角是全等的,所以該多面體具有具有高度鏡射和旋轉對稱。
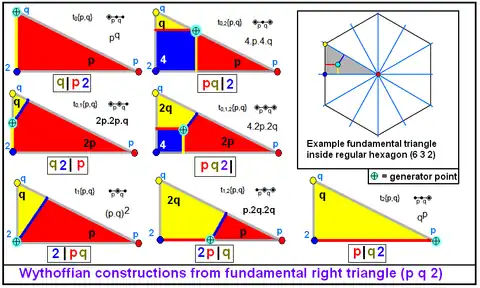
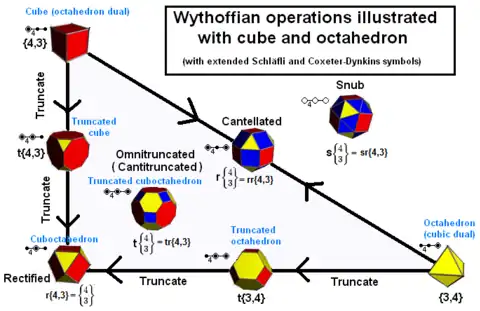
Example forms from the cube and octahedron
均勻多面體可能是正多面體(同時具備面可遞、邊可遞)、擬正多面體(若邊可遞,則面不可遞)或半正多面體(邊未必可遞面也未必可遞)。由於面和頂角不一定要是凸的,所以很多均勻多面體的也是星狀多面體。
不包括無限集合,有75個均勻多面體(如果允許邊緣重合則有76種)。
參考文獻
- Brückner, M. Vielecke und vielflache. Theorie und geschichte.. Leipzig, Germany: Teubner, 1900.
- Coxeter, Harold Scott MacDonald; Longuet-Higgins, M. S.; Miller, J. C. P. . Philosophical Transactions of the Royal Society of London. Series A. Mathematical and Physical Sciences (The Royal Society). 1954, 246 (916): 401–450. ISSN 0080-4614. JSTOR 91532. MR 0062446. doi:10.1098/rsta.1954.0003.
- Sopov, S. P. . Ukrainskiui Geometricheskiui Sbornik. 1970, (8): 139–156. MR 0326550.
- Wenninger, Magnus. . Cambridge University Press. 1974. ISBN 0-521-09859-9.
- Skilling, J. . Philosophical Transactions of the Royal Society of London. Series A. Mathematical and Physical Sciences. 1975, 278 (1278): 111–135. ISSN 0080-4614. JSTOR 74475. MR 0365333. doi:10.1098/rsta.1975.0022.
- Har'El, Z. Uniform Solution for Uniform Polyhedra., Geometriae Dedicata 47, 57-110, 1993. Zvi Har’El, Kaleido software, Images, dual images
- Mäder, R. E.(页面存档备份,存于) Uniform Polyhedra. Mathematica J. 3, 48-57, 1993. (页面存档备份,存于)
- Messer, Peter W. Closed-Form Expressions for Uniform Polyhedra and Their Duals., Discrete & Computational Geometry 27:353-375 (2002).
外部連結
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.