度数矩阵

给定一个图 ${\displaystyle G=(V,E)}$ 与 ${\displaystyle |V|=n}$， ${\displaystyle G}$的度数矩阵 ${\displaystyle D}$是一个 ${\displaystyle n\times n}$的对角线矩阵，其定义为[1]

${\displaystyle d_{i,j}:=\left\{{\begin{matrix}\deg(v_{i})&{\mbox{if}}\ i=j\\0&{\mbox{otherwise}}\end{matrix}}\right.}$

其中度数 ${\displaystyle \deg(v_{i})}$为这个顶点上的边的条数。 在无向图中，这意味着每个环会使得度数增加2。 在有向图中，术语“度”可以指“入度”（indegree，终点在这个顶点的边的条数）或“出度”（ outdegree，起点在这个顶点的边的条数）。

下面的无向图有一个6x6的度数矩阵，其数值为。

Vertex labeled graph	度数矩阵
	${\displaystyle {\begin{pmatrix}4&0&0&0&0&0\\0&3&0&0&0&0\\0&0&2&0&0&0\\0&0&0&3&0&0\\0&0&0&0&3&0\\0&0&0&0&0&1\\\end{pmatrix}}}$

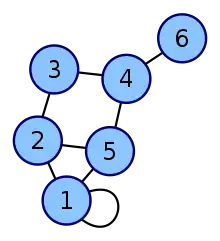注意，对于无向图而言，开始和结束于同一节点的边（即环，如上图中的节点1）会使相应的度增加2（即被计算两次）。

一个k-正则图的度数矩阵是恒为${\displaystyle k}$的对角线矩阵。

根据度的总和公式，度数矩阵的迹是对应图的边数的两倍。