正扭歪無限面體
歷史
關於考克斯特,1926年時,約翰·弗林德斯·皮特里將扭歪多邊形(非平面多邊形)的概念推廣到四維空間的扭歪多面體和三維空間的扭歪無限面體。
考克斯特找到了三種形式,他們具有平的面和扭歪的頂點圖,兩者彼此互補。它們都可以用施萊夫利符號的擴展符號{l,m|n}來表示。這個擴展符號{l,m|n}表示每個頂點都是個正邊形的公共頂點,且存在正邊形的空洞。
若一扭歪無限面體是一個正扭歪無限面體,則其施萊夫利符號存在下列等式:
- 2 sin(π/l) · sin(π/m) = cos(π/n)
三維空間的正扭歪無限面體
三維空間中有三種扭歪無限面體,分別為四角六片四角孔扭歪無限面體、六角四片四角孔扭歪無限面體和六角六片三角孔扭歪無限面體。約翰·康威將他們稱為多立方體(英語:)、多八面體(英語:)和、多四面體(英語:),英文中的字首mu-表示「多」(英語:)的意思,其意義分別代表「很多立方體」、「很多八面體」以及「很多四面體」[2]。
- 四角六片四角孔扭歪無限面體(多立方體、英語:):{4,6|4}:每個頂點都是六個正方形的公共頂點
- 六角四片四角孔扭歪無限面體(多八面體、英語:):{6,4|4}:每個頂點都是四個六邊形的公共頂點
- 六角六片三角孔扭歪無限面體(多四面體、英語:):{6,6|3}:每個頂點都是六個六邊形的公共頂點
考克斯特給予這些 {2q,2r|p} 形式的扭歪無限面體與抽象群 (2q,2r|2,p) 同構的[[(p,q,p,r)]+的手徵對稱性。與之相關的堆砌就具有[[(p,q,p,r)]]的擴展對稱性[3]。
| 考克斯特群 對稱性 |
無限面體 {p,q|l} | 圖像 | 面 {p} | 洞 {l} | 頂點圖 | 相關堆砌 | |
|---|---|---|---|---|---|---|---|
[[4,3,4]] [[4,3,4]+] |
{4,6|4} 四角六片四角孔 扭歪無限面體 多立方體 |  動畫 |  |  | 扭歪六邊形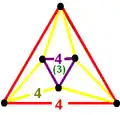 (黃色部分) | t0,3{4,3,4} |  |
| {6,4|4} 六角四片四角孔 扭歪無限面體 多八面體 |  動畫 |  | 扭歪四邊形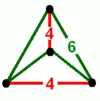 (綠色部分) | 2t{4,3,4} | 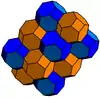 | ||
[[3[4]]] [[3[4]]+] | {6,6|3} 六角六片三角孔 扭歪無限面體 多四面體 |  動畫 |  |  | 扭歪六邊形 (綠色部分) | q{4,3,4} | 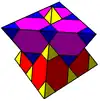 |
三維雙曲空間的正扭歪無限面體
1967年時,C. W. L. Garner以類似於在歐式三維空間尋找正扭歪無限面體的方式,發現了31種雙曲空間中具有扭歪多邊形頂點圖的正扭歪無限面體[4]。
14種緊空間正扭歪無限面體
| 考克斯特群 | 無限面體 {p,q|l} | 面 {p} | 洞 {l} | 堆砌 | 頂點圖 | 無限面體 {p,q|l} | 面 {p} | 洞 {l} | 堆砌 | 頂點圖 | |
|---|---|---|---|---|---|---|---|---|---|---|---|
[3,5,3] | {10,4|3} |  |  | 2t{3,5,3} |  | {4,10|3} |  |  | t0,3{3,5,3} |  | |
[5,3,5] | {6,4|5} |  |  | 2t{5,3,5} |  | {4,6|5} |  |  | t0,3{5,3,5} |  | |
[(4,3,3,3)] | {8,6|3} |  |  | ct{(4,3,3,3)} |  | {6,8|3} |  |  | ct{(3,3,4,3)} |  | |
[(5,3,3,3)] | {10,6|3} |  |  | ct{(5,3,3,3)} |  | {6,10|3} |  |  | ct{(3,3,5,3)} | 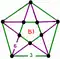 | |
[(4,3,4,3)] | {8,8|3} |  |  | ct{(4,3,4,3)} |  | {6,6|4} |  |  | ct{(3,4,3,4)} | 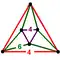 | |
[(5,3,4,3)] | {8,10|3} |  |  | ct{(4,3,5,3)} | 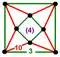 | {10,8|3} |  |  | ct{(5,3,4,3)} |  | |
[(5,3,5,3)] | {10,10|3} |  |  | ct{(5,3,5,3)} |  | {6,6|5} |  |  | ct{(3,5,3,5)} |  |
17種仿緊空間正扭歪無限面體
| 考克斯特群 | 無限面體 {p,q|l} | 面 {p} | 洞 {l} | 堆砌 | 頂點圖 | 無限面體 {p,q|l} | 面 {p} | 洞 {l} | 堆砌 | 頂點圖 | |
|---|---|---|---|---|---|---|---|---|---|---|---|
[4,4,4] | {8,4|4} |  |  | 2t{4,4,4} |  | {4,8|4} |  |  | t0,3{4,4,4} |  | |
[3,6,3] | {12,4|3} |  |  | 2t{3,6,3} |  | {4,12|3} |  |  | t0,3{3,6,3} |  | |
[6,3,6] | {6,4|6} |  |  | 2t{6,3,6} |  | {4,6|6} |  |  | t0,3{6,3,6} |  | |
[(4,4,4,3)] | {8,6|4} |  |  | ct{(4,4,3,4)} |  | {6,8|4} |  |  | ct{(3,4,4,4)} |  | |
[(4,4,4,4)] | {8,8|4} |  |  | q{4,4,4} |  | ||||||
[(6,3,3,3)] | {12,6|3} |  |  | ct{(6,3,3,3)} |  | {6,12|3} |  |  | ct{(3,3,6,3)} |  | |
[(6,3,4,3)] | {12,8|3} |  |  | ct{(6,3,4,3)} |  | {8,12|3} |  |  | ct{(4,3,6,3)} |  | |
[(6,3,5,3)] | {12,10|3} |  |  | ct{(6,3,5,3)} |  | {10,12|3} |  |  | ct{(5,3,6,3)} |  | |
[(6,3,6,3)] | {12,12|3} |  |  | ct{(6,3,6,3)} |  | {6,6|6} |  |  | ct{(3,6,3,6)} |  |
參考文獻
- . gigazine.net. 2014-02-22 [2016-07-16]. (原始内容存档于2020-11-19).
- The Symmetry of Things, 2008, Chapter 23 Objects with Primary Symmmetry, Infinite Platonic Polyhedra, pp. 333–335
- Coxeter, Regular and Semi-Regular Polytopes II 2.34)
- Garner, C. W. L. Regular Skew Polyhedra in Hyperbolic Three-Space. Canad. J. Math. 19, 1179–1186, 1967. (页面存档备份,存于) Note: His paper says there are 32, but one is self-dual, leaving 31.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.