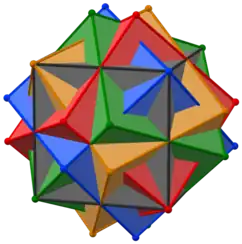
五複合立方體

在幾何學中，五複合立方體，是一種由五個立方體組合成的複合多面體，其索引編號為UC₉，是唯一五種正複合體之一[3]，亦是一種星形多面體。埃德蒙·赫斯在1876年首先描述了該幾何結構。

構造

擁有二十面體對稱五複合立方體可以由以原點為中心、面向軸的第一個立方體開始構造，其餘的立方體則透過軸 $(1,\phi ,0)$ 旋轉 $-{\frac {2n\pi }{5}}$ 弧度來構造，畢依這加入順序決定角度值中的n，例如第二個立方體n=1、第三個立方體n=2以此類推。

五複合立方體為五個立方體組合成的形狀，因此其邊、面和頂點的數量基本上應該會是立方體的5倍，但因為部分頂點是重合的，因此其僅有30個面、60條邊和20個頂點。

五複合立方體中可以找到菱形三十面體中的30個菱形[4][5]。

五複合立方體可以視為正十二面體刻面後的多面體，在正十二面體凸包中每個立方體定位在12個頂點中的其中8個頂點。

由於五複合立方體可以看作是在正十二面體中嵌入立方體，因此其頂點座標與正十二面體相同：

(±1, ±1, ±1)

(0, ±1ϕ, ±ϕ)

(±1ϕ, ±ϕ, 0)

(±ϕ, 0, ±1ϕ)

其中ϕ = 1 + √5/2為黃金比例。

五複合立方體可以看作是一種菱形三十面體的星形多面體，即星形菱形三十面體[6][7]。

星狀圖	星形	星狀核	凸包
		菱形三十面體	正十二面體

五複合立方體的凸包是正十二面體。其與一些凸包也是正十二面體的多面體有著相同的稜排佈，例如小雙三斜三十二面體、大雙三斜三十二面體和雙三斜十二面體。

亦有其他也由五個立方體組合成的形狀，例如佛達里也斯的五複合立方體。這種形狀是一個八面體對稱的星形多面體。

Cromwell, Peter R., , Cambridge, 1997. p 360
Harman, Michael G., , unpublished manuscript, c. 1974 [2016-09-01], （原始内容存档于2013-07-31）.
Skilling, John, , Mathematical Proceedings of the Cambridge Philosophical Society, 1976, 79: 447–457, MR 0397554, doi:10.1017/S0305004100052440.
Cundy, H. and Rollett, A. "Five Cubes in a Dodecahedron." §3.10.6 in Mathematical Models, 3rd ed. Stradbroke, England: Tarquin Pub., pp. 135–136, 1989.

H.S.M. Coxeter, Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8
Regular Polytopes (1973)[1] pp.49-50, p.98
Regular Polytopes (1973)[1], 3.6 The five regular compounds, pp.47-50
Steinhaus, H. Mathematical Snapshots, 3rd ed. New York: Dover, 1999. ISBN 978-0486409146 p. 199
Ball, W. W. R. and Coxeter, H. S. M. Mathematical Recreations and Essays, 13th ed. New York: Dover, pp. 135 and 137, 1987. ISBN 978-0486253572
Weisstein, Eric W. (编). . at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. （英语）.
Kabai, S. Mathematical Graphics I: Lessons in Computer Graphics Using Mathematica. Püspökladány, Hungary: Uniconstant, pp. 161 and 185, 2002.

This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.