2i
是在虛數軸正向距離原點兩個單位的純虛數,屬於高斯整數[2]:13,為虛數單位的兩倍[2]:12,同時也是負四的平方根[2]:12[3][4]:ix[5][6][7][8],是方程式的正虛根[3][9]:10。日常生活中通常不會用來計量事物,例如無法具體地描述何謂個人,邏輯上個人並沒有意義。[10]部分書籍或教科書偶爾會使用來做虛數的例子或題目。[11]
| 2i | |
|---|---|
| 數表 — 高斯整數 << −3i −2i −i 0 i 2i 3i >> | |
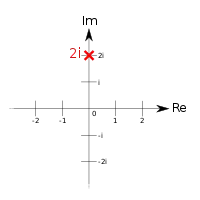 在高斯平面上的位置 | |
| 命名 | |
| 名稱 | 2i 負四的平方根 二虛數單位 |
| 性質 | |
| 高斯整數分解 | |
| 絕對值 | 2[1] |
| 以此為根的多項式或函數 | |
| 表示方式 | |
| 值 | 2倍虛數單位 |
| 代數形式 | |
| 十进制 | 2i |
| -1+i进制 | 1110100 |
| 2i进制 | 10 |
| 高斯整數導航 | ||||||
|---|---|---|---|---|---|---|
| ↑ | ||||||
| 2i | ||||||
| −1+i | i | 1+i | ||||
| ← | −2 | −1 | 0 | 1 | 2 | → |
| −1−i | −i | 1−i | ||||
| −2i | ||||||
| ↓ | ||||||
在高斯平面上,與相鄰的高斯整數有和(上下相鄰;純虛數)以及和(左右相鄰),然而複數不具備有序性,即無法判斷與間的大小關係,因此無法定義與何者為的前一個虛數、何者為的下一個虛數。
| −1+3i | 3i | 1+3i |
| −1+2i | 2i | 1+2i |
|---|---|---|
| −1+i | i | 1+i |
| 與相鄰的高斯整數示意圖 | ||
性質
- 不屬於實數,是一個純虛數,同時也是複數位於複數平面,其實部為0、虛部為2[12],輻角為90度(弧度)[13],其也能表達為[14]:7或[15]。
- 是一個高斯整數[16][17][18],高斯整數分解為[19]:711,或[20]:433,其中,1+i為2i的高斯質因數。[19]:711[21][22]:247
- 所有複數的可以表達為之冪的線性組合。[23]換句話說若進位制以為底數,則可獨一無二地表示全體複數[24]。該進制稱為2i進制,由高德納於1955年發現。[25]
- 複數的虛數部可以定義為複數與其共軛複數之差除以的商,[26]換言之,則。[2]:32
- 正弦函數可以定義為純虛指數函數與其倒數之差除以的商。[27][28]:41[2]:64
- 等於最小的質數和虛數單位的積,即[15],其中為第個質數。
- 虛數單位和虛數單位的倒數相差。
- 任意數與相乘的意義為模放大兩倍並在複平面上以原點為中心逆時針旋轉90度。[14]:7[2]:20-21
相關數字
−2i
是的相反數,其平方根曾提議作為複數進位制的底數。[36]
1+i
是的平方根[28],同時也是高斯質數[37]。由於其冪次為1+i、 2i、 −2+2i、 −4、 −4−4i、 −8i...,在正負、虛實交替變化,因此若作為進位制底數可以表達全體複數。但其組合變化相較於為底數的進位制,做為底數更為適合。[38]亦有另外一個數也為的平方根,即,但這個數較少出現於探討進位制底數的文章中,也沒有其他特殊的性質。此外,也不是第一象限高斯質數,因此鮮少被拿出來討論。
−1+i
| −1+i | |
|---|---|
| 命名 | |
| 名稱 | −1+i |
| 性質 | |
| 高斯整數分解 | |
| 絕對值 | |
| 表示方式 | |
| 值 | |
| 代數形式 | |
| 十进制 | −1+i |
| 2i进制 | 113.2 |
是的平方根。距離原點單位,輻角為135度(弧度[39]),其實部為負一、虛部為1。不是高斯質數,其可以分解為i和1+i的乘積。由於其冪次為−1+i、 −2i、 2+2i、 −4、 4−4i、 8i...,其在正負、虛實交替變化,因此其可以構建一個以為底數並用1和0表達複數的進位制[36][40]。其他複數雖然也可以,如,但對高斯整數而言,以為底並不是一個優良的選擇。[38]雖然也是的平方根,但因為上述原因,所以這個數字通不會用來作為進制的底數來使用。
除了外,其他形式的複數也能作為進位制底數,但其在表達數的範圍不同,以為例,其表達的範圍較為均勻,而、等則會越來越狹長。[41]
| 十進制 | 二進制 | 2i進制 | −1+i進制 | −2+i進制 |
|---|---|---|---|---|
| 0 | 0 | 0 | 0 | 0 |
| 1 | 1 | 1 | 1 | 1 |
| 2 | 10 | 2 | 1100 | 2 |
| −1 | −1 | 103 | 11101 | 144 |
| −2 | −10 | 102 | 11100 | 143 |
| i | i | 10.2 | 11 | 12 |
| 2i | 10i | 10 | 1110100 | 24 |
| 3i | 11i | 20.2 | 1110111 | 1341 |
| −i | −i | 0.2 | 111 | 133 |
| −2i | −10i | 1030 | 100 | 121 |
| −3i | −11i | 1030.2 | 110011 | 13304 |
| 1+i | 1+i | 11.2 | 1110 | 13 |
| 1−i | 1−i | 1.2 | 111010 | 134 |
| −1+i | −1+i | 113.2 | 10 | 11 |
| −1−i | −1−i | 103.2 | 110 | 132 |
| 2+i | 10+i | 12.2 | 1111 | 14 |
| 2−i | 10−i | 2.2 | 111011 | 1440 |
| −2+i | −10+i | 112.2 | 11111 | 10 |
| −2−i | −10−i | 102.2 | 11101011 | 131 |
相鄰的高斯整數
| −1+3i | 3i | 1+3i |
| −1+2i | 2i | 1+2i |
|---|---|---|
| −1+i | i | 1+i |
| 與相鄰的高斯整數示意圖 | ||
參考文獻
- . geeksforgeeks.org. [2022-09-15]. (原始内容存档于2022-09-15).
- Mokhithi, Mashudu and Shock, Jonathan, (PDF), Jonathan Shock, 2020 [2022-06-23], (原始内容存档 (PDF)于2022-07-04)
- . themathpage.com. [2022-06-23]. (原始内容存档于2022-07-13).
- Hart, P. . iUniverse. 2008 [2022-06-23]. ISBN 9780595435036. (原始内容存档于2022-08-20).
- . sciencefocus.com. 2019-06-21 [2022-06-23]. (原始内容存档于2022-07-12).
- Williams, Travis D. . The Palgrave Handbook of Literature and Mathematics (Springer). 2021: 399–418.
- Neuman, Yrsa. . Wittgenstein and the Limits of Language (Routledge). 2019: 159–171.
- Parker, Barry. . Chaos in the Cosmos (Springer). 1996: 129–154.
- (PDF). people.math.wisc.edu. [2022-06-23]. (原始内容存档 (PDF)于2022-01-21).
- Abubakr, Mohammed. . arXiv preprint arXiv:1101.2798. 2011 [2022-06-23]. doi:10.48550/ARXIV.1101.2798. (原始内容存档于2022-07-04).
- 中學數學實用詞典, 九章出版社, 孫文先, P.22 中的示範其解為2i, ISBN 957-603-093-5
- (PDF). ichthyosapiens.com. [2022-06-23]. (原始内容存档 (PDF)于2017-08-29).
- . mathway.com. [2022-06-23]. (原始内容存档于2022-06-25).
- Jeremy Orloff. (PDF). math.mit.edu. [2022-06-23]. (原始内容存档 (PDF)于2021-11-03).
- Wolfram, Stephen. . from Wolfram Alpha: Computational Knowledge Engine, Wolfram Research (英语).
- C. F. Gauss, Theoria residuorum biquadraticorum. Commentatio secunda., Comm. Soc. Reg. Sci. Gottingen 7 (1832) 1-34; reprinted in Werke, Georg Olms Verlag, Hildesheim, 1973, pp. 93-148.
- 从数到环:环论的早期历史 (页面存档备份,存于),由Israel Kleiner所作 (Elem. Math. 53 (1998) 18 – 35)
- Ribenboim, Paulo, , New York: Springer, 1996, ISBN 0-387-94457-5
- Banerjee, Ashmi and Mukherjee, Shaunak and Datta, Somjit and Majumder, Subhashis. . 2015 International Conference on Control Communication & Computing India (ICCC) (IEEE). 2015: 710–715 [2022-08-15]. (原始内容存档于2022-08-15).
- Briggs, WE. . The Mathematics Teacher (National Council of Teachers of Mathematics). 1966, 59 (5): 432–436.
- Kerins, Bowen. . The Mathematics Teacher (National Council of Teachers of Mathematics). 2003, 96 (5): 350–357.
- Gallian, Joseph A and Jungreis, Douglas S. . The American Mathematical Monthly (Taylor & Francis). 1988, 95 (3): 247–249.
- Blest, David C and Jamil, Tariq. . International Journal of Mathematical Education in Science and Technology (Taylor & Francis). 2003, 34 (4): 561–574.
- Donald Knuth. . Communications of the ACM. April 1960, 3 (4).
- D. Knuth. The Art of Computer Programming. Volume 2, 3rd Edition. Addison-Wesley. pp. 205, "Positional Number Systems"
- (PDF), hawaii.edu, [2022-06-23], (原始内容存档 (PDF)于2022-06-26)
- Weisstein, Eric W. (编). . at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).
- Bak, Joseph and Newman, Donald J and Newman, Donald J, (PDF) 8, Springer, 2010 [2022-06-23], (原始内容存档 (PDF)于2022-06-25)
- Robert Braunwart. . School Science and Mathematics. 1965-04, 65 (4): 292–295 [2022-06-23]. doi:10.1111/j.1949-8594.1965.tb13422.x. (原始内容存档于2022-06-27) (英语).
- Sloane, N.J.A. (编). . The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- Sloane, N.J.A. (编). . The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- Sloane, N.J.A. (编). . The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- Sarkar, Souradip and Gomony, Manil Dev. . 2018 Design, Automation & Test in Europe Conference & Exhibition (DATE) (IEEE). 2018: 1481–1483 [2022-08-15]. (原始内容存档于2022-08-20).
- Dujella, Andrej. . Glasnik Matematicki (HRVATSKO MATEMATICKO DRUSTVO). 1997, 32: 1–10.
- Collins, George E. . Journal of Symbolic Computation (Elsevier). 2002, 33 (4): 385–392.
- Penney, Walter. (PDF). J. ACM. 1965, 12 (2): 247–248 [2022-06-23]. (原始内容存档 (PDF)于2022-07-04).
- Sven Simon. . The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- Gilbert, William J. (PDF). The Mathematical Intelligencer (Springer). 1982, 4 (2): 78–86 [2022-06-23]. (原始内容存档 (PDF)于2022-01-02).
- . from Wolfram Alpha: Computational Knowledge Engine, Wolfram Research.
- Gilbert, William J. . Mathematics Magazine (Taylor & Francis). 1984, 57 (2): 77–81.
- Gilbert, William J. (PDF). Canadian mathematical bulletin (Cambridge University Press). 1986, 29 (4): 495–500 [2022-08-20]. (原始内容存档 (PDF)于2022-08-20).