無限胞體
在幾何學中，無限胞體或無限胞形是指有無限多個胞或維面的多胞體。其在數學上可以分成兩大類：[1]
另外一個相關議題為無限維多胞體，然而相關研究領域尚未成熟，因此學術上尚未有一個對無限維多胞體的普遍接受之定義。[2][3]
種類
無限胞體（英語：）意指有無限個面、無限個胞、無限條邊和無限個頂點的多胞體。
其性質皆與無限面體相似，由空間密鋪即空間堆砌組成。四维空間的正無限胞體只有一種，即立方體堆砌[4]。
| 維度 | 三維 退化四維 |
四維 退化五維 | |
|---|---|---|---|
| 图像 |  立方體堆砌 |
 超立方體堆砌 |
 十六胞體堆砌 |
| 施萊夫利符號 | {4,3,4} | {4,3,3,4} | {3,3,4,3} |
於雙曲空間亦的對應的幾何結構：
| 圖像 |  六階四面體堆砌 |
 五階立方體堆砌 |
 四階八面體堆砌 |
 四階十二面體堆砌 |
 三階二十面體堆砌 |
|---|---|---|---|---|---|
| 六階四面體堆砌 | 五階立方體堆砌 | 四階八面體堆砌 | 四階十二面體堆砌 | 三階二十面體堆砌 | |
| 施萊夫利符號 | {3,3,6} | {4,3,5} | {3,4,4} | {5,3,4} | {3,5,3} |
五維雙曲空間也有三種正無限胞體：
| 名稱 | 五階正五胞體堆砌 | 五階超立方體堆砌 | 四階二十四胞體堆砌 | 三階一百二十胞體堆砌 |
|---|---|---|---|---|
| 無限胞體的胞 |  正五胞體 |
 超立方體 |
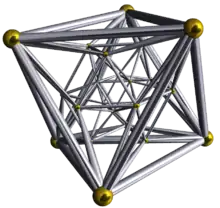 正二十四胞體 |
 正一百二十胞體 |
| 正五胞體 | 超立方體 | 正二十四胞體 | 正一百二十胞體 | |
| 施萊夫利符號 | {3,3,3,5} | {4,3,3,5} | {3,4,3,4} | {5,3,3,3} |
二維空間
三維空間
三維空間中的扭歪無限胞體即扭歪無限面體，目前已知有三種正圖形屬於此類：
| 三維空間中的正扭歪無限面體的局部 | ||
|---|---|---|
 四角六片四角孔扭歪無限面體 {4,6|4} |
 六角四片四角孔扭歪無限面體 {6,4|4} |
 六角六片三角孔扭歪無限面體 {6,6|3} |
另外亦有30種正無限面體存於三維歐氏空間[6]。
參考文獻
- Grünbaum, B.; "Regular Polyhedra—Old and New", Aeqationes mathematicae, Vol. 16 (1977), pp 1–20.
- Phelphs, R. R. . MATHEMATICA SCANDINAVICA. 1969, 24: 5–26. doi:10.7146/math.scand.a-10917.
- Maserick, P. H. . Illinois J. Math. 1965, 9 (no. 4): 623––635. doi:10.1215/ijm/1256059305.
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, (2008) The Symmetries of Things, ISBN 978-1-56881-220-5 (Chapter 21, Naming the Archimedean and Catalan polyhedra and tilings, Architectonic and Catoptric tessellations, p 292-298, includes all the nonprismatic forms)
- Lagarias, J. C.; Moews, D., , Discrete and Computational Geometry, 1995, 13 (3–4): 573–583, MR 1318797, doi:10.1007/BF02574064.
- McMullen & Schulte (2002，Section 7E)
參考書目
- McMullen, Peter; Schulte, Egon,
, Encyclopedia of Mathematics and its Applications 92, Cambridge: Cambridge University Press, 2002, ISBN 0-521-81496-0, MR 1965665, doi:10.1017/CBO9780511546686
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.