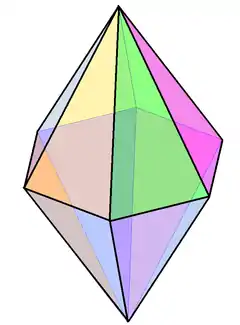
雙七角錐
在幾何學中,雙七角錐是指以七邊形做為基底的雙錐體。所有雙七角錐都有14個面,21個邊和9個頂點[1][2]。所有雙七角錐都是十四面體。
 | |||
| 類別 | 雙錐體 | ||
|---|---|---|---|
| 對偶多面體 | 七角柱 | ||
| 數學表示法 | |||
| 考克斯特符號 | |||
| 施萊夫利符號 | { } + {7} | ||
| 康威表示法 | dP7 | ||
| 性質 | |||
| 面 | 14 | ||
| 邊 | 21 | ||
| 頂點 | 9 | ||
| 歐拉特徵數 | F=14, E=21, V=9 (χ=2) | ||
| 組成與佈局 | |||
| 面的種類 | 14個三角形(側面) 基底為七邊形 | ||
| 面的佈局 | V4.4.7 | ||
| 對稱性 | |||
| 對稱群 | D7h, [7,2], (*227), order 28 | ||
| 旋轉對稱群 | D7, [7,2]+, (227), order 14 | ||
| 特性 | |||
| 凸 | |||
| 圖像 | |||
| |||
如果雙七角錐以正七邊形做為基底則可稱為雙正七角錐或正七角雙錐。每個面都是正多邊形的正七角雙錐不存在,因為正六角雙錐已經是平面了,每個面都是正多邊形的正七角雙錐將會變成七階三角形鑲嵌的一部分,因此正七角雙錐不是半正多面體。其在施萊夫利符號中用{ } + {7}表示,具有D7與D7h對稱群。
正七角雙錐能在自然界中存在,例如某些化學結構[3],如九硼離子B9-有一種分子異構形為正七角雙錐[4]、有機金屬錯合物[(C7H7)V(CO)3]也具有正七角雙錐結構[5]。
相關多面體與鑲嵌
| 對稱群:[7,2], (*722) | [7,2]+, (722) | ||||||||
|---|---|---|---|---|---|---|---|---|---|
 |
 |
 |
 |
 | |||||
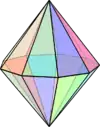
| {7,2} | t{7,2} | r{7,2} | 2t{7,2}=t{2,7} | 2r{7,2}={2,7} | rr{7,2} | tr{7,2} | sr{7,2} | ||
| 半正對偶 | |||||||||
 |
 |
 |
 |
 |
 |
 | |||
| V72 | V142 | V72 | V4.4.7 | V27 | V4.4.7 | V4.4.14 | V3.3.3.7 | ||
| 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | ... | ∞ |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
 |
 |
 |
 |
 |
 |
|||||||
| 作为球面镶嵌 | ||||||||||||
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
||
參見
參考文獻
- Heptagonal Dipyramid (页面存档备份,存于) dmccooey.com [2014-6-23]
- Pugh, Anthony, , University of California Press: 21, 27, 62, 1976 [2014-06-23], ISBN 9780520030565, (原始内容存档于2014-07-09).
- Marcel Gielen, Rudolph Willem, Bernd Wrackmeyer, Fluxional Organometallic and Coordination Compounds,Physical Organometallic Chemistry, John Wiley & Sons, 2005, ISBN 9780470858448, p20
- Pan, Li-Li, Jun Li, and Lai-Sheng Wang. "Low-lying isomers of the B9- boron cluster: The planar molecular wheel versus three-dimensional structures." The Journal of chemical physics 129.2 (2008): 024302.
- Florian P. Pruchnik, Organometallic Chemistry of the Transition Elements, Modern Inorganic Chemistry, Springer, 1990 ,ISBN 9780306431920, PT127
外部連結
- 埃里克·韦斯坦因. . MathWorld.
- Olshevsky, George, Bipyramid at Glossary for Hyperspace.
- Virtual Reality Polyhedra (页面存档备份,存于) The Encyclopedia of Polyhedra
- VRML models <7>
- Conway Notation for Polyhedra (页面存档备份,存于) Try: dP7
- John Montroll A Plethora of Polyhedra in Origami p38 Courier Dover Publications, 2002,ISBN 9780486422718 正七角雙錐的摺法
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.

